Sketch the graph of \(f\).

Find the values of \(x\) where the function is decreasing.

The function \(f\) can also be written in the form \(f(x) = a\sin \left( {\frac{\pi }{4}(x + c)} \right)\), where \(a \in \mathbb{R}\), and \(0 \leqslant c \leqslant 2\). Find the value of \(a\);

The function \(f\) can also be written in the form \(f(x) = a\sin \left( {\frac{\pi }{4}(x + c)} \right)\), where \(a \in \mathbb{R}\), and \(0 \leqslant c \leqslant 2\). Find the value of \(c\).

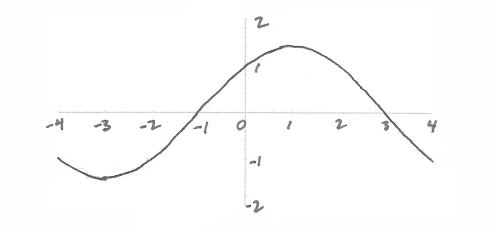          A1A1A1     N3

Note:     Award A1 for approximately correct sinusoidal shape.

     Only if this A1 is awarded, award the following:

     A1 for correct domain,

     A1 for approximately correct range.

[3 marks]

recognizes decreasing to the left of minimum or right of maximum,

eg     \(f'(x) < 0\)     (R1)

x-values of minimum and maximum (may be seen on sketch in part (a))     (A1)(A1)

eg     \(x =  - 3,{\text{ (1, 1.4)}}\)

two correct intervals     A1A1     N5

eg     \( - 4 < x <  - 3,{\text{ }}1 \leqslant x \leqslant 4;{\text{ }}x <  - 3,{\text{ }}x \geqslant 1\)

[5 marks]

recognizes that \(a\) is found from amplitude of wave     (R1)

y-value of minimum or maximum     (A1)

eg     (−3, −1.41) , (1, 1.41)

\(a = 1.41421\)

\(a = \sqrt 2 {\text{,   (exact), 1.41,}}\)     A1     N3

[3 marks]

METHOD 1

recognize that shift for sine is found at x-intercept     (R1)

attempt to find x-intercept    (M1)

eg     \(\cos \left( {\frac{\pi }{4}x} \right) + \sin \left( {\frac{\pi }{4}x} \right) = 0,{\text{ }}x = 3 + 4k,{\text{ }}k \in \mathbb{Z}\)

\(x =  - 1\)     (A1)

\(c = 1\)     A1     N4

METHOD 2

attempt to use a coordinate to make an equation     (R1)

eg     \(\sqrt 2 \sin \left( {\frac{\pi }{4}c} \right) = 1,{\text{ }}\sqrt 2 \sin \left( {\frac{\pi }{4}(3 - c)} \right) = 0\)

attempt to solve resulting equation     (M1)

eg     sketch, \(x = 3 + 4k,{\text{ }}k \in \mathbb{Z}\)

\(x =  - 1\)     (A1)

\(c = 1\)     A1     N4

[4 marks]