| Date | May 2008 | Marks available | 5 | Reference code | 08M.1.sl.TZ2.1 |
| Level | SL only | Paper | 1 | Time zone | TZ2 |
| Command term | Find | Question number | 1 | Adapted from | N/A |
Question
A box contains 100 cards. Each card has a number between one and six written on it. The following table shows the frequencies for each number.
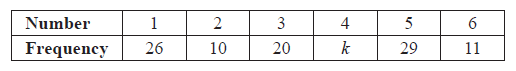
Calculate the value of k.
Find
(i) the median;
(ii) the interquartile range.
Markscheme
evidence of using ∑fi=100 (M1)
k=4 A1 N2
[2 marks]
(i) evidence of median position (M1)
e.g. 50th item, 26+10+20=56
median=3 A1 N2
(ii) Q1=1 and Q3=5 (A1)(A1)
interquartile range=4 (accept 1 to 5 or 5−1 , etc.) A1 N3
[5 marks]
Examiners report
Frequencies and median seemed well understood, but quartiles and inter-quartile range less so.
Frequencies and median seemed well-understood, but quartiles and interquartile range less so. A few students, probably based on past papers, drew cumulative frequency diagrams, generating slightly different answers for median and quartiles.

