| Date | November 2014 | Marks available | 1 | Reference code | 14N.2.SL.TZ0.1 |
| Level | Standard level | Paper | Paper 2 | Time zone | Time zone 0 |
| Command term | Calculate | Question number | 1 | Adapted from | N/A |
Question
Data analysis question.
A student sets up a circuit to study the variation of resistance R of a negative temperature coefficient (NTC) thermistor with temperature T. The data are shown plotted on the graph.
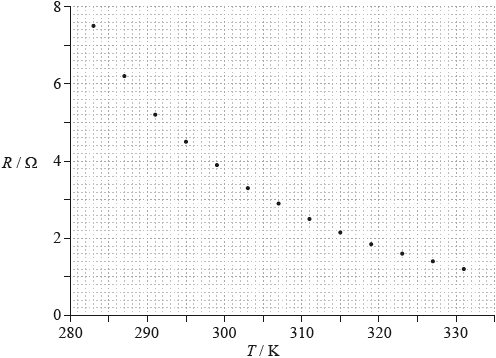
The electric current through the thermistor for T=283 K is 0.78 mA. The uncertainty in the electric current is 0.01 mA.
Draw the best-fit line for the data points.
Calculate the gradient of the graph when T=291 K.
State the unit for your answer to (b)(i).
The uncertainty in the resistance value is 5%. The uncertainty in the temperature is negligible. On the graph, draw error bars for the data point at T=283 K and for the data point at T=319 K.
Calculate the power dissipated by the thermistor at T=283 K.
Determine the uncertainty in the power dissipated by the thermistor at T=283 K.
Markscheme
smooth curve that passes within ±0.5 squares of all data points;
a tangent drawn at [291, 5.2] and selection of two extreme points on the tangent that use ΔR>3.5 Ω; } (judge by eye)
gradient magnitude determined as 0.20±0.02;
negative value given;
Ω K−1;
correct error bar for 283 K (total length of bar 3–5 squares, centred on point);
correct error bar for 319 K (total length of bar 0.5–2 squares, centred on point);
substituting I2R=([0.78×10−3]2×7.5)=4.5×10−6 Wor4.6×10−6 W;
fractional uncertainty in I2=2×0.010.78 (= 0.026or2.6%);
uncertainty in power (=[0.026+0.05]×4.6×10−6)=0.34×10−6 W to 0.35×10−6 W;
answer rounded to 1 significant figure;
or
uncertainty in I2=2×1.3%/0.026;
total uncertainty in P=7.6%/0.076;
answer rounded to 1 significant figure;
Examiners report
Very poorly executed. Few SL candidates knew how to draw an acceptable tangent.

