| Date | November 2015 | Marks available | 2 | Reference code | 15N.2.HL.TZ0.1 |
| Level | Higher level | Paper | Paper 2 | Time zone | Time zone 0 |
| Command term | Suggest | Question number | 1 | Adapted from | N/A |
Question
Data analysis question.
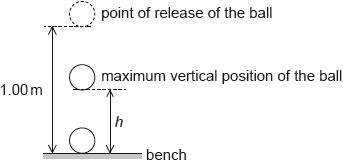
An experiment is undertaken to investigate the relationship between the temperature of a ball and the height of its first bounce.
A ball is placed in a beaker of water until the ball and the water are at the same temperature. The ball is released from a height of 1.00 m above a bench. The maximum vertical height h from the bottom of the ball above the bench is measured for the first bounce. This procedure is repeated twice and an average hmean is calculated from the three measurements.
The procedure is repeated for a range of temperatures. The graph shows the variation of hmean with temperature T.
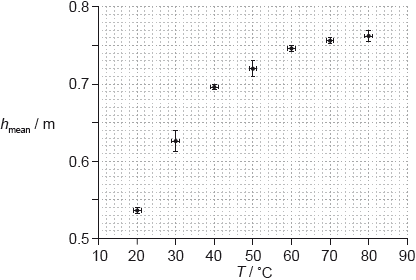
A student hypothesizes that hmean is proportional to T2.
Comment, using two points on your line of best-fit, whether or not this is a valid hypothesis.
Suggest why using two points cannot confirm that hmean is proportional to T2.
The temperature is measured using a liquid in glass thermometer. Explain why it is likely that the uncertainty in T is constant.
Markscheme
coordinates of two points on the line correctly read from the graph; } (check points read to within half a square and ignore omission of powers of ten in reading)
T2h or hT2 calculated for both values;
consistent conclusion that values similar within the (typical) experimental error so sensible / differ outside (typical) experimental error so not sensible;} (must see reference to experimental error not just bald statement)
Award [2 max] for a graph of hmean versus T2 and a conclusion that hypothesis is not valid.
Do not award credit for “does not go through origin”.
two points define a straight line / any arbitrary curve can pass through two points; to confirm hypothesis third point (or more) must lie on the straight line;
or
refers to experimental error in data (and therefore error in ratio) / depending on the two points chosen the hypothesis may be confirmed;
increasing the number of data points increases the strength of conclusion;
or
one of the two points chosen may be anomalous/erroneous/outlier;
third point needed to confirm hypothesis;
same thermometer used;
same eyes used;
same reading method used;
this type of thermometer has (typically) equal graduations;
liquid in thermometer expands linearly;
Examiners report
Many read two points correctly from the line, but too often examiners saw lines that missed a data point with the printed point still being used for the read-off. These derived data then generally led to a correct evaluation of hT2 (or it’s reciprocal). However, for full marks, the examiners needed to see some consideration of the (sometimes considerable) error represented by the error bars and this was only rarely present.
There were a number of alternative statements that could gain credit here. The most frequently seen suggestion was that, because two points can define a line of any curvature, therefore a third (or more) data point is required to establish the proportionality.

