| Date | November 2012 | Marks available | 1 | Reference code | 12N.2.sl.TZ0.3 |
| Level | SL | Paper | 2 | Time zone | TZ0 |
| Command term | State | Question number | 3 | Adapted from | N/A |
Question
Chemical equilibrium and kinetics are important concepts in chemistry.
The oxidation of sulfur dioxide is an important reaction in the Contact process used to manufacture sulfuric acid.
2SO2(g)+O2(g)⇌2SO3(g)ΔH=−198.2 kJ
Vanadium(V) oxide, V2O5, is a catalyst that can be used in the Contact process. It provides an alternative pathway for the reaction, lowering the activation energy, Ea.
A glass container is half-filled with liquid bromine and then sealed. The system eventually reaches a dynamic equilibrium. State one characteristic of a system in equilibrium.
(i) Deduce the equilibrium constant expression, Kc.
(ii) Predict how each of the following changes affects the position of equilibrium and
the value of Kc.
(i) Define the term activation energy, Ea.
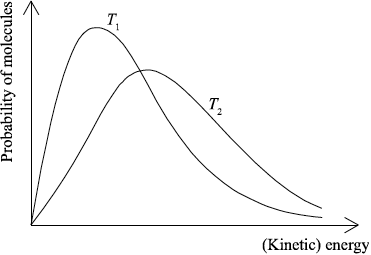
(ii) Sketch the two Maxwell–Boltzmann energy distribution curves for a fixed amount of gas at two different temperatures, T1 and T2 (T2>T1). Label both axes.

Markscheme
rate of forward process/reaction = rate of backwards/reverse process/reaction / rate of vaporization/evaporation = rate of condensation;
concentrations of reactants and products remain constant;
no change in macroscopic properties / closed system / constant matter/energy / OWTTE;
Do not accept concentration of reactants and products are equal.
Accept constant colour of Br2 vapour/liquid.
(i) (Kc=)[SO3]2[SO2]2[O2];
(ii) 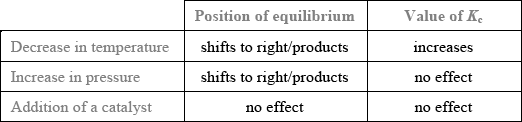
Award [1] for any two or three correct, [2] for any four or five correct, [3] for six correct.
(i) minimum/least/smallest energy needed (by reactants/colliding particles) to react/start/initiate a reaction;
Allow energy difference between reactants and transition state.
(ii) x-axis label: (kinetic) energy/(K)E and y-axis label: probability/fraction of molecules/particles / probability density;
Allow number of molecules/particles for y-axis.
correct shape of a typical Maxwell–Boltzmann energy distribution curve;
Do not award mark if curve is symmetric, does not start at zero or if it crosses x-axis.
two curves represented with second curve for T2>T1 to right of first curve, peak maximum lower than first curve and after the curves cross going to the right, T2 curve needs to be above T1 curve as illustrated;
M2 and M3 can be scored independently.
Examiners report
The equilibrium question was generally well answered, but some candidates suggested that the forward reaction equal the reverse reaction without reference to the rates, while some other candidates incorrectly stated that the concentration of reactants and products are equal.
In part (b) (i), the Kc expression was usually written correctly. Part (b) (ii) was done well and candidates showed a good understanding of the effect of temperature and catalyst on an equilibrium system; however, weaker candidates incorrectly identified a change in the value of Kc on increasing the pressure.
In part (c), the word minimum was often missed in the definition of activation energy. In the Maxwell–Boltzmann energy distribution curves, many candidates labelled the axes incorrectly. Also in some cases, the curves did not start at the origin or the curve for T2 was drawn incorrectly at the same level on the y-axis. The weaker students drew an enthalpy level diagram instead of a Maxwell- Boltzmann distribution.

