Find the general solution of the differential equation, expressing your answer in the form \(f(x,{\text{ }}y) = c\), where \(c\) is a constant.

(i)     Hence find the particular solution passing through the points \((1,{\rm{  \pm }}\sqrt 2 )\).

(ii)     Sketch the graph of your solution and name the type of curve represented.

(i)     Write down the particular solution passing through the points \((1,{\text{ }} \pm 1)\).

(ii)     Give a geometrical interpretation of this solution in relation to part (b).

(i)     Find the general solution of the differential equation \(\frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{x}{y} + \frac{y}{x}\), where \(xy \ne 0\).

(ii)     Find the particular solution passing through the point \((1,{\text{ }}\sqrt 2 )\).

(iii)     Sketch the particular solution.

(iv)     The graph of the solution only contains points with \(\left| x \right| > a\).

Find the exact value of \(a,{\text{ }}a > 0\).

attempt to separate the variables     M1

\(\int {y\frac{{{\text{d}}y}}{{{\text{d}}x}}{\text{d}}x = \int {x{\text{d}}x} } \)    A1

Note:     Accept \(\int {y{\text{d}}y = \int {x{\text{d}}x} } \).

obtain \(\frac{1}{2}{y^2} = \frac{1}{2}{x^2} + {\text{ constant }}( \Rightarrow {y^2} - {x^2} = c)\)     A1

[3 marks]

(i)     substitute the coordinates for both points     M1

\({( \pm \sqrt 2 )^2} - {1^2} = 1\)

obtain \({y^2} - {x^2} = 1\) or equivalent     A1

(ii) 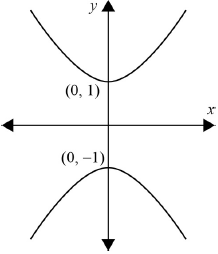         A1A1

Note:     A1 for general shape including two branches and symmetry;

A1 for values of the intercepts.

(rectangular) hyperbola     A1

[5 marks]

(i)     \({y^2} - {x^2} = 0\)     A1

(ii)     the two straight lines \(y =  \pm x\)     A1

form the asymptotes to the hyperbola found above, or equivalent     A1

[3 marks]

(i)     the equation is homogeneous, so attempt to substitute \(y = vx\)     M1

as a first step write \(\frac{{{\text{d}}y}}{{{\text{d}}x}} = x\frac{{{\text{d}}v}}{{{\text{d}}x}} + v\)     (A1)

then \(x\frac{{{\text{d}}v}}{{{\text{d}}x}} + v = \frac{1}{v} + v\)     A1

attempt to solve the resulting separable equation     M1

\(\int {v{\text{d}}v = \int {\frac{1}{x}{\text{d}}x} } \)    A1

obtain \(\frac{1}{2}{v^2} = \ln \left| x \right| + {\text{ constant}} \Rightarrow {y^2} = 2{x^2}\ln \left| x \right| + c{x^2}\)     A1

(ii)     substituting the coordinates     (M1)

obtain \(c = 2 \Rightarrow {y^2} = 2{x^2}\ln \left| x \right| + 2{x^2}\)     A1

(iii)          A1

(iv)     since \({y^2} > 0\) and \({x^2} \ne 0\)     R1

\(\ln \left| x \right| >  - 1 \Rightarrow \left| x \right| > {{\text{e}}^{ - 1}}\)    A1

\(a = {{\text{e}}^{ - 1}}\)    A1

Note:     The R1 may be awarded for a correct reason leading to subsequent correct work.

[12 marks]

Although (a), (b) and (c) were generally well done, it was rare to see a completely satisfactory geometrical answer to part (c)(ii). A few candidates solved the differential equation as a homogeneous equation.

Although (a), (b) and (c) were generally well done, it was rare to see a completely satisfactory geometrical answer to part (c)(ii). A few candidates solved the differential equation as a homogeneous equation.

Although (a), (b) and (c) were generally well done, it was rare to see a completely satisfactory geometrical answer to part (c)(ii). A few candidates solved the differential equation as a homogeneous equation.

For part (d) most candidates used the correct solution method for a homogeneous differential equation. A few found the algebra hard going in finding the particular solution. Most approaches to the final part were unsatisfactory, with a lack of proper consideration of the inequalities in the question.