| Date | May 2017 | Marks available | 2 | Reference code | 17M.1.sl.TZ2.12 |
| Level | SL only | Paper | 1 | Time zone | TZ2 |
| Command term | Find | Question number | 12 | Adapted from | N/A |
Question
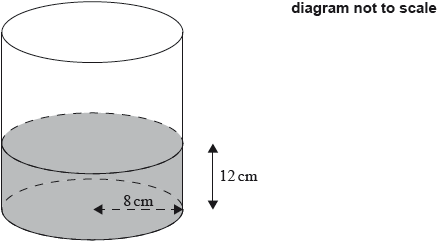
A cylindrical container with a radius of 8 cm is placed on a flat surface. The container is filled with water to a height of 12 cm, as shown in the following diagram.
A heavy ball with a radius of 2.9 cm is dropped into the container. As a result, the height of the water increases to h cm, as shown in the following diagram.
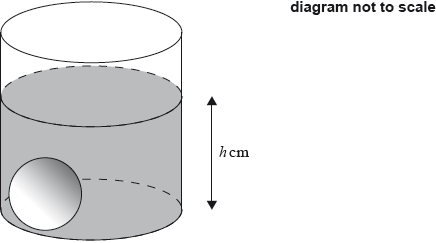
Find the volume of water in the container.
Find the value of h.
Markscheme
π×82×12 (M1)
Note: Award (M1) for correct substitution into the volume of a cylinder formula.
2410 cm3 (2412.74… cm3, 768π cm3) (A1) (C2)
[2 marks]
43π×2.93+768π=π×82h (M1)(M1)(M1)
Note: Award (M1) for correct substitution into the volume of a sphere formula (this may be implied by seeing 102.160…), (M1) for adding their volume of the ball to their part (a), (M1) for equating a volume to the volume of a cylinder with a height of h.
OR
43π×2.93=π×82(h−12) (M1)(M1)(M1)
Note: Award (M1) for correct substitution into the volume of a sphere formula (this may be implied by seeing 102.160…), (M1) for equating to the volume of a cylinder, (M1) for the height of the water level increase, h−12. Accept h for h−12 if adding 12 is implied by their answer.
(h=) 12.5 (cm) (12.5081… (cm)) (A1)(ft) (C4)
Note: If 3 sf answer used, answer is 12.5 (12.4944…). Follow through from part (a) if first method is used.
[4 marks]

