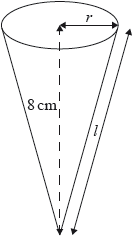
Find the radius, $r$, of the circular base of the cone.

Find the slant height, $l$, of the cone.

Find the curved surface area of the cone.

$100 = \frac{1}{3}\pi {r^2}(8)$     (M1)

Note:     Award (M1) for correct substitution into volume of cone formula.

$r = 3.45{\text{ (cm) }}\left( {3.45494 \ldots {\text{ (cm)}}} \right)$     (A1)     (C2)

[2 marks]

${l^2} = {8^2} + {(3.45494 \ldots )^2}$     (M1)

Note:     Award (M1) for correct substitution into Pythagoras’ theorem.

$l = 8.71{\text{ (cm) }}\left( {8.71416 \ldots {\text{ (cm)}}} \right)$     (A1)(ft)     (C2)

Note:     Follow through from part (a).

[2 marks]

$\pi  \times 3.45494 \ldots  \times 8.71416 \ldots$     (M1)

Note:     Award (M1) for their correct substitutions into curved surface area of a cone formula.

$= 94.6{\text{ c}}{{\text{m}}^2}{\text{ }}(94.5836 \ldots {\text{ c}}{{\text{m}}^2})$     (A1)(ft)     (C2)

Note:     Follow through from parts (a) and (b). Accept $94.4{\text{ c}}{{\text{m}}^2}$ from use of 3 sf values.

[2 marks]