| Date | November 2015 | Marks available | 2 | Reference code | 15N.1.sl.TZ0.10 |
| Level | SL only | Paper | 1 | Time zone | TZ0 |
| Command term | Find | Question number | 10 | Adapted from | N/A |
Question
Let y=f(x), for −0.5≤ x ≤ 6.5. The following diagram shows the graph of f′, the derivative of f.
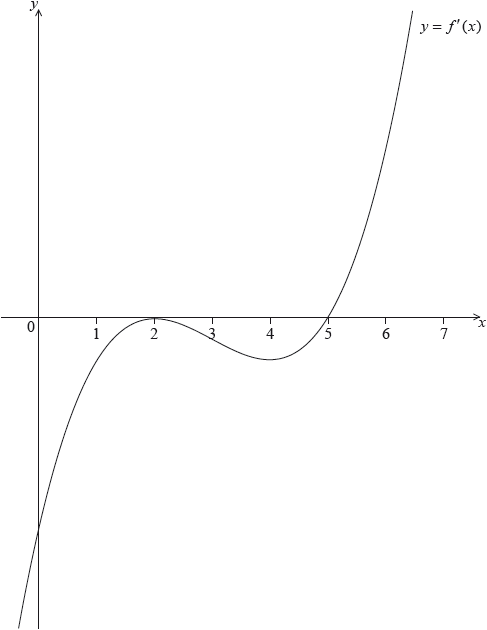
The graph of f′ has a local maximum when x=2, a local minimum when x=4, and it crosses the x-axis at the point (5, 0).
Explain why the graph of f has a local minimum when x=5.
Find the set of values of x for which the graph of f is concave down.
The following diagram shows the shaded regions A, B and C.
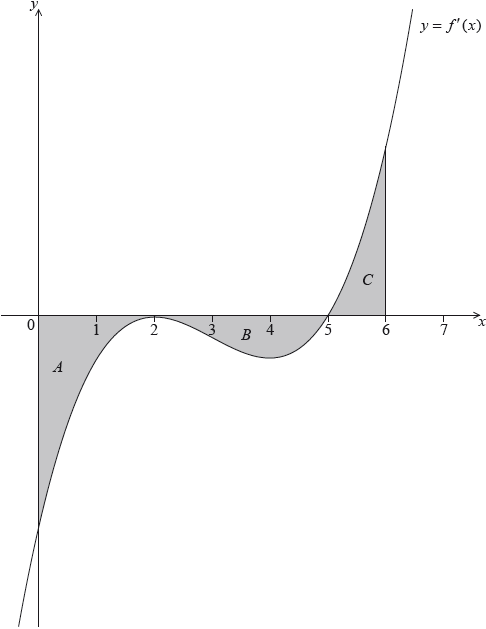
The regions are enclosed by the graph of f′, the x-axis, the y-axis, and the line x=6.
The area of region A is 12, the area of region B is 6.75 and the area of region C is 6.75.
Given that f(0)=14, find f(6).
The following diagram shows the shaded regions A, B and C.

The regions are enclosed by the graph of f′, the x-axis, the y-axis, and the line x=6.
The area of region A is 12, the area of region B is 6.75 and the area of region C is 6.75.
Let g(x)=(f(x))2. Given that f′(6)=16, find the equation of the tangent to the graph of g at the point where x=6.
Markscheme
METHOD 1
f′(5)=0 (A1)
valid reasoning including reference to the graph of f′ R1
egf′ changes sign from negative to positive at x=5, labelled sign chart for f′
so f has a local minimum at x=5 AG N0
Note: It must be clear that any description is referring to the graph of f′, simply giving the conditions for a minimum without relating them to f′ does not gain the R1.
METHOD 2
f′(5)=0 A1
valid reasoning referring to second derivative R1
egf″(5)>0
so f has a local minimum at x=5 AG N0
[2 marks]
attempt to find relevant interval (M1)
egf′ is decreasing, gradient of f′ is negative, f″<0
2<x<4(accept “between 2 and 4”) A1 N2
Notes: If no other working shown, award M1A0 for incorrect inequalities such as 2≤ x ≤ 4, or “from 2 to 4”
[2 marks]
METHOD 1 (one integral)
correct application of Fundamental Theorem of Calculus (A1)
eg∫60f′(x)dx=f(6)−f(0), f(6)=14+∫60f′(x)dx
attempt to link definite integral with areas (M1)
eg∫60f′(x)dx=−12−6.75+6.75, ∫60f′(x)dx=Area A+Area B+ Area C
correct value for ∫60f′(x)dx (A1)
eg∫60f′(x)dx=−12
correct working A1
egf(6)−14=−12, f(6)=−12+f(0)
f(6)=2 A1 N3
METHOD 2 (more than one integral)
correct application of Fundamental Theorem of Calculus (A1)
eg∫20f′(x)dx=f(2)−f(0), f(2)=14+∫20f′(x)
attempt to link definite integrals with areas (M1)
eg∫20f′(x)dx=12, ∫52f′(x)dx=−6.75, ∫60f′(x)=0
correct values for integrals (A1)
eg∫20f′(x)dx=−12, ∫25f′(x)dx=6.75, f(6)−f(2)=0
one correct intermediate value A1
egf(2)=2, f(5)=−4.75
f(6)=2 A1 N3
[5 marks]
correct calculation of g(6) (seen anywhere) A1
eg22, g(6)=4
choosing chain rule or product rule (M1)
egg′(f(x))f′(x), dydx=dydu×dudx, f(x)f′(x)+f′(x)f(x)
correct derivative (A1)
egg′(x)=2f(x)f′(x), f(x)f′(x)+f′(x)f(x)
correct calculation of g′(6) (seen anywhere) A1
eg2(2)(16), g′(6)=64
attempt to substitute their values of g′(6) and g(6) (in any order) into equation of a line (M1)
eg22=(2×2×16)6+b, y−6=64(x−4)
correct equation in any form A1 N2
egy−4=64(x−6), y=64x−380
[6 marks]
[Total 15 marks]

