| Date | November 2012 | Marks available | 4 | Reference code | 12N.1.sl.TZ0.4 |
| Level | SL only | Paper | 1 | Time zone | TZ0 |
| Command term | Find | Question number | 4 | Adapted from | N/A |
Question
Part of the graph of f(x)=ax3−6x2f(x)=ax3−6x2 is shown below.
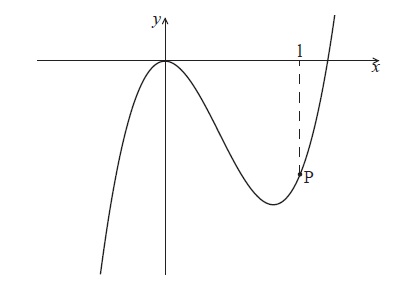
The point P lies on the graph of ff . At P, x = 1.
Find f′(x) .
The graph of f has a gradient of 3 at the point P. Find the value of a .
Markscheme
f′(x)=3ax2−12x A1A1 N2
Note: Award A1 for each correct term.
[2 marks]
setting their derivative equal to 3 (seen anywhere) A1
e.g. f′(x)=3
attempt to substitute x=1 into f′(x) (M1)
e.g. 3a(1)2−12(1)
correct substitution into f′(x) (A1)
e.g. 3a−12 , 3a=15
a=5 A1 N2
[4 marks]
Examiners report
A majority of candidates answered part (a) correctly, and a good number earned full marks on both parts of this question. In part (b), some common errors included setting the derivative equal to zero, or substituting 3 for x in their derivative. There were also a few candidates who incorrectly tried to work with f(x) , rather than f′(x) , in part (b).
A majority of candidates answered part (a) correctly, and a good number earned full marks on both parts of this question. In part (b), some common errors included setting the derivative equal to zero, or substituting 3 for x in their derivative. There were also a few candidates who incorrectly tried to work with f(x) , rather than f′(x) , in part (b).

