| Date | May 2015 | Marks available | 3 | Reference code | 15M.1.sl.TZ1.9 |
| Level | SL only | Paper | 1 | Time zone | TZ1 |
| Command term | Show that | Question number | 9 | Adapted from | N/A |
Question
A function f has its derivative given by f′(x)=3x2−2kx−9, where k is a constant.
Find f″.
The graph of f has a point of inflexion when x = 1.
Show that k = 3.
Find f'( - 2).
Find the equation of the tangent to the curve of f at ( - 2,{\text{ }}1), giving your answer in the form y = ax + b.
Given that f'( - 1) = 0, explain why the graph of f has a local maximum when x = - 1.
Markscheme
f''(x) = 6x - 2k A1A1 N2
[2 marks]
substituting x = 1 into f'' (M1)
eg\;\;\;f''(1),{\text{ }}6(1) - 2k
recognizing f''(x) = 0\;\;\;(seen anywhere) M1
correct equation A1
eg\;\;\;6 - 2k = 0
k = 3 AG N0
[3 marks]
correct substitution into f'(x) (A1)
eg\;\;\;3{( - 2)^2} - 6( - 2) - 9
f'( - 2) = 15 A1 N2
[2 marks]
recognizing gradient value (may be seen in equation) M1
eg\;\;\;a = 15,{\text{ }}y = 15x + b
attempt to substitute ( - 2,{\text{ }}1) into equation of a straight line M1
eg\;\;\;1 = 15( - 2) + b,{\text{ }}(y - 1) = m(x + 2),{\text{ }}(y + 2) = 15(x - 1)
correct working (A1)
eg\;\;\;31 = b,{\text{ }}y = 15x + 30 + 1
y = 15x + 31 A1 N2
[4 marks]
METHOD 1 ({{\text{2}}^{{\text{nd}}}} derivative)
recognizing f'' < 0\;\;\;(seen anywhere) R1
substituting x = - 1 into f'' (M1)
eg\;\;\;f''( - 1),{\text{ }}6( - 1) - 6
f''( - 1) = - 12 A1
therefore the graph of f has a local maximum when x = - 1 AG N0
METHOD 2 ({{\text{1}}^{{\text{st}}}} derivative)
recognizing change of sign of f'(x)\;\;\;(seen anywhere) R1
eg\;\;\;sign chart\;\;\;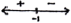
correct value of f' for - 1 < x < 3 A1
eg\;\;\;f'(0) = - 9
correct value of f' for x value to the left of - 1 A1
eg\;\;\;f'( - 2) = 15
therefore the graph of f has a local maximum when x = - 1 AG N0
[3 marks]
Total [14 marks]
Examiners report
Well answered and candidates coped well with k in the expression.
Mostly answered well with the common error being to substitute into f' instead of f''.
A straightforward question that was typically answered correctly.
Some candidates recalculated the gradient, not realising this had already been found in part c). Many understood they were finding a linear equation but were hampered by arithmetic errors.
Using change of sign of the first derivative was the most common approach used with a sign chart or written explanation. However, few candidates then supported their approach by calculating suitable values for f'(x). This was necessary because the question already identified a local maximum, hence candidates needed to explain why this was so. Some candidates did not mention the ‘first derivative’ just that ‘it’ was increasing/decreasing. Few candidates used the more efficient second derivative test.

