| Date | May 2017 | Marks available | 1 | Reference code | 17M.2.sl.TZ2.2 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Write down | Question number | 2 | Adapted from | N/A |
Question
The maximum temperature T, in degrees Celsius, in a park on six randomly selected days is shown in the following table. The table also shows the number of visitors, N, to the park on each of those six days.
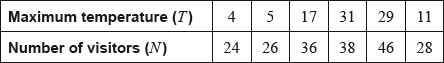
The relationship between the variables can be modelled by the regression equation N=aT+b.
Find the value of a and of b.
Write down the value of r.
Use the regression equation to estimate the number of visitors on a day when the maximum temperature is 15 °C.
Markscheme
evidence of set up (M1)
egcorrect value for a or b
0.667315, 22.2117
a=0.667, b=22.2 A1A1 N3
[3 marks]
0.922958
r=0.923 A1 N1
[1 marks]
valid approach (M1)
eg0.667(15)+22.2, N(15)
32.2214 (A1)
32 (visitors) (must be an integer) A1 N2
[3 marks]

