| Date | May 2015 | Marks available | 4 | Reference code | 15M.2.sl.TZ2.3 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Find and Write down | Question number | 3 | Adapted from | N/A |
Question
The following table shows the sales, y millions of dollars, of a company, x years after it opened.
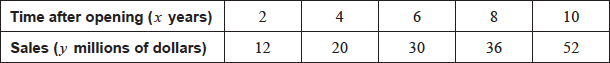
The relationship between the variables is modelled by the regression line with equation y=ax+b.
(i) Find the value of a and of b.
(ii) Write down the value of r.
Hence estimate the sales in millions of dollars after seven years.
Markscheme
(i) evidence of set up (M1)
egcorrect value for a, b or r
a=4.8, b=1.2 A1A1 N3
(ii) r=0.988064
r=0.988 A1 N1
[4 marks]
correct substitution into their regression equation (A1)
eg4.8×7+1.2
34.8 (millions of dollars) (accept 35 and 34800000) A1 N2
[2 marks]
Total [6 marks]
Examiners report
Many answered this question completely correct, showing familiarity with the GDC operation for finding the equation of the line and coefficient. It was not uncommon to see a=5.05 and b=−0.488, which indicates incorrect use of the GDC lists to find the values.
Some candidates attempted an algebraic approach to finding the regression line and a few seemed to not recognize that r represents the coefficient of correlation.
Many answered this question completely correct, showing familiarity with the GDC operation for finding the equation of the line and coefficient. It was not uncommon to see a=5.05 and b=−0.488, which indicates incorrect use of the GDC lists to find the values.
Some candidates attempted an algebraic approach to finding the regression line and a few seemed to not recognize that r represents the coefficient of correlation.

