| Date | None Specimen | Marks available | 2 | Reference code | SPNone.2.sl.TZ0.8 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Interpret | Question number | 8 | Adapted from | N/A |
Question
Each day, a factory recorded the number ( x ) of boxes it produces and the total production cost ( y ) dollars. The results for nine days are shown in the following table.
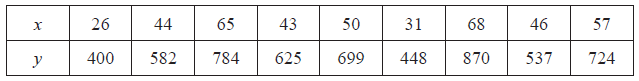
Write down the equation of the regression line of y on x .
Use your regression line from part (a) as a model to answer the following.
Interpret the meaning of
(i) the gradient;
(ii) the y-intercept.
Estimate the cost of producing 60 boxes.
The factory sells the boxes for $19.99 each. Find the least number of boxes that the factory should produce in one day in order to make a profit.
Comment on the appropriateness of using your model to
(i) estimate the cost of producing 5000 boxes;
(ii) estimate the number of boxes produced when the total production cost is $540.
Markscheme
y=10.7x+121 A1A1 N2
[2 marks]
(i) additional cost per box (unit cost) A1 N1
(ii) fixed costs A1 N1
[2 marks]
attempt to substitute into regression equation M1
e.g. y=10.7×60+121 , y=760.12…
cost=$760 (accept $763 from 3 s.f. values) A1 N2
[2 marks]
setting up inequality (accept equation) M1
e.g. 19.99x>10.7x+121
x>12.94… A1
13 boxes (accept 14 from x>13.02 , using 3 s.f. values) A1 N2
Note: Exception to the FT rule: if working shown, award the final A1 for a correct integer solution for their value of x.
[3 marks]
(i) this would be extrapolation, not appropriate R1R1 N2
(ii) this regression line cannot predict x from y, not appropriate R1R1 N2
[4 marks]

