| Date | May 2015 | Marks available | 3 | Reference code | 15M.2.sl.TZ1.1 |
| Level | SL only | Paper | 2 | Time zone | TZ1 |
| Command term | Predict | Question number | 1 | Adapted from | N/A |
Question
The following table shows the average number of hours per day spent watching television by seven mothers and each mother’s youngest child.
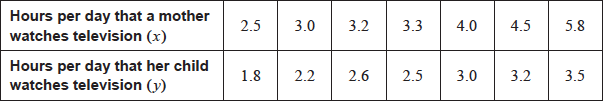
The relationship can be modelled by the regression line with equation y=ax+b.
(i) Find the correlation coefficient.
(ii) Write down the value of a and of b.
Elizabeth watches television for an average of 3.7 hours per day.
Use your regression line to predict the average number of hours of television watched per day by Elizabeth’s youngest child. Give your answer correct to one decimal place.
Markscheme
(i) evidence of valid approach (M1)
eg1 correct value for r, (or for a or b, seen in (ii))
0.946591
r=0.947 A1 N2
(ii) a=0.500957, b=0.803544
a=0.501, b=0.804 A1A1 N2
[4 marks]
substituting x=3.7 into their equation (M1)
eg0.501(3.7)+0.804
2.65708(2 hours 39.4252 minutes) (A1)
y=2.7 (hours) (must be correct 1 dp, accept 2 hours 39.4 minutes) A1 N3
[3 marks]
Total [7 marks]
Examiners report
Candidates continue to have difficulty using their GDCs to find and correctly identify the coefficients of a linear regression. Both the r and r2 values were often given as candidates were hedging their bets and were not entirely clear which one to give. Candidates frequently were unable to find the correct values for a and b suggesting a lack of familiarity working with GDCs. It was also surprising to see so many candidates leave these values to only one significant figure sacrificing all the marks for this part. Subsequent use of their line to find y for a given x was not difficult for most, but answers were not often given to the required accuracy of one decimal place.
Candidates continue to have difficulty using their GDCs to find and correctly identify the coefficients of a linear regression. Both the r and r2 values were often given as candidates were hedging their bets and were not entirely clear which one to give. Candidates frequently were unable to find the correct values for a and b suggesting a lack of familiarity working with GDCs. It was also surprising to see so many candidates leave these values to only one significant figure sacrificing all the marks for this part. Subsequent use of their line to find y for a given x was not difficult for most, but answers were not often given to the required accuracy of one decimal place.

