DP Mathematics: Analysis and Approaches Questionbank

Show that $\frac{\text{d}y}{\text{d}x}=\frac{y \cos \left(xy\right)}{2y-x \cos \left(xy\right)}$.

Prove that, when $\frac{\text{d}y}{\text{d}x}=0 , y=\pm 1$.

Hence find the coordinates of all points on $C$, for $0<x<4\mathrm{\pi }$, where $\frac{\text{d}y}{\text{d}x}=0$.

Show that $\frac{dy}{dx}=\frac{3x^2}{2{\text{e}}^{2y}-1}$.

The tangent to $C$ at the point Ρ is parallel to the $y$-axis.

Find the $x$-coordinate of Ρ.

Use the differential equation $\frac{dy}{dx}=\frac{y^2+3xy+2x^2}{x^2}$ to show that the points of zero gradient on the curve lie on two straight lines of the form $y=mx$ where the values of $m$ are to be determined.

The curve has a point of inflexion at $\left(x_1, y_1\right)$ where ${\text{e}}^{-\frac{\mathrm{\pi }}{2}}<x_1<{\text{e}}^{\frac{\mathrm{\pi }}{2}}$. Determine the coordinates of this point of inflexion.

At time $T$, the following conditions are true.

Boat $\text{B}$ has travelled $10$ metres further than boat $\text{A}$.
Boat $\text{B}$ is travelling at double the speed of boat $\text{A}$.
The rate of change of the angle $\theta$ is $-0.1$ radians per second.

Find the speed of boat $\text{A}$ at time $T$.

Hence find the equation of the tangent to $C$ at the point where $x=1$.

Show that $\frac{dy}{dx}+\left(x\frac{{\displaystyle dy}}{{\displaystyle dx}}+y\right)\left(1+\ln \left(xy\right)\right)=1$.

By substituting $Y=\frac{dy}{dt}$, show that $Y=B{\text{e}}^{2t}$ where $B$ is a constant.

By solving the differential equation $\frac{dy}{dt}=y$, show that $y=A{\text{e}}^t$ where $A$ is a constant.

Show that $\frac{dx}{dt}-x=-A{\text{e}}^t$.

Hence find $y$ as a function of $t$.

Show that $\frac{d^{2}y}{d{t}^2}-2\frac{dy}{dt}-3y=0$.

Hence show that $x=-\frac{B}{2}{\text{e}}^{2t}+C$, where $C$ is a constant.

Find the two values for $\lambda$ that satisfy $\frac{d^{2}y}{d{t}^2}-2\frac{dy}{dt}-3y=0$.

Let the two values found in part (c)(ii) be ${\lambda }_1$ and ${\lambda }_2$.

Verify that $y=F{\text{e}}^{{\lambda }_{1}t}+G{\text{e}}^{{\lambda }_{2}t}$ is a solution to the differential equation in (c)(i),where $G$ is a constant.

Solve the differential equation in part (a)(ii) to find $x$ as a function of $t$.

By differentiating $\frac{dy}{dt}=-x+y$ with respect to $t$, show that $\frac{d^{2}y}{d{t}^2}=2\frac{{\displaystyle dy}}{{\displaystyle dt}}$.

Find the equation of the tangent to $C$ at $\text{P}$.

Show that $\frac{dy}{dx}=\pm \frac{3x^2+1}{2\sqrt{x^3+x}}$, for $x>0$.

Find the time it takes to fill the container to its maximum volume.

Find the rate of change of the height of the water when the container is filled to half its maximum volume.

Find an expression for the volume of water $V({\text{m}}^3)$ in the trough in terms of $\theta$.

Calculate $\frac{\text{d}\theta }{\text{d}t}$ when $\theta =\frac{\pi }{3}$.

Find $\frac{\text{d}y}{\text{d}x}$ in terms of $x$ and $y$.

Determine the equation of the tangent to $C$ at the point $\left(\frac{2}{\text{e}},\text{ e}\right)$

Show that $\frac{\text{d}y}{\text{d}x}=-\left(\frac{1+y\text{sin}\left(xy\right)}{1+x\text{sin}\left(xy\right)}\right)$.

Find the coordinates of P and Q.

Given that the gradients of the tangents to C at P and Q are m₁ and m₂ respectively, show that m₁ × m₂ = 1.

Find the coordinates of the three points on C, nearest the origin, where the tangent is parallel to the line $y=-x$.

Using implicit differentiation, or otherwise, find $\frac{\text{d}y}{\text{d}x}$ for each curve in terms of $x$ and $y$.

Let P($a$, $b$) be the unique point where the curves $C_1$ and $C_2$ intersect.

Show that the tangent to $C_1$ at P is perpendicular to the tangent to $C_2$ at P.

Find an expression for $\frac{\text{d}y}{\text{d}x}$ in terms of $x$ and $y$.

Find the equations of the tangents to this curve at the points where the curve intersects the line $x=1$.

Find the coordinates of the points on the curve $y^3+3x{y}^2-x^3=27$ at which $\frac{\text{d}y}{\text{d}x}=0$.

The folium of Descartes is a curve defined by the equation $x^3+y^3-3xy=0$, shown in the following diagram.

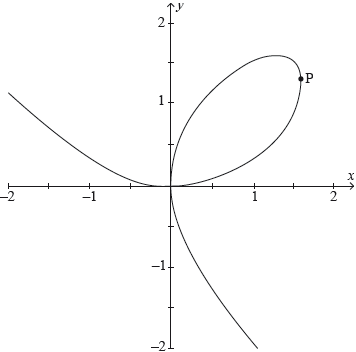
Determine the exact coordinates of the point P on the curve where the tangent line is parallel to the $y$-axis.

Using implicit differentiation, find an expression for $\frac{\text{d}y}{\text{d}x}$.

Find the equation of the tangent to the curve at the point $\left(\frac{1}{4}\text{, }\frac{5\pi }{6}\right)$.

A camera at point C is 3 m from the edge of a straight section of road as shown in the following diagram. The camera detects a car travelling along the road at $t$ = 0. It then rotates, always pointing at the car, until the car passes O, the point on the edge of the road closest to the camera.

A car travels along the road at a speed of 24 ms⁻¹. Let the position of the car be X and let OĈX = θ.

Find $\frac{\text{d}\theta }{\text{d}t}$, the rate of rotation of the camera, in radians per second, at the instant the car passes the point O .