Sketch the graph of $y = f(x)$, indicating clearly any asymptotes and points of intersection with the $x$ and $y$ axes.

Find an expression for ${f^{ - 1}}(x)$.

Find all values of $x$ for which $f(x) = {f^{ - 1}}(x)$.

Solve the inequality $\left| {f(x)} \right| < \frac{3}{2}$.

Solve the inequality $f\left( {\left| x \right|} \right) < \frac{3}{2}$.

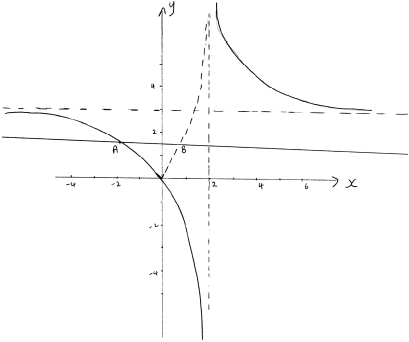
Note: In the diagram, points marked $A$ and $B$ refer to part (d) and do not need to be seen in part (a).

shape of curve     A1

Note:     This mark can only be awarded if there appear to be both horizontal and vertical asymptotes.

intersection at $(0,{\text{ }}0)$     A1

horizontal asymptote at $y = 3$     A1

vertical asymptote at $x = 2$     A1

[4 marks]

$y = \frac{{3x}}{{x - 2}}$

$xy - 2y = 3x$     M1A1

$xy - 3x = 2y$

$x = \frac{{2y}}{{y - 3}}$

$\left( {{f^{ - 1}}(x)} \right) = \frac{{2x}}{{x - 3}}$     M1A1

Note:     Final M1 is for interchanging of $x$ and $y$, which may be seen at any stage.

[4 marks]

METHOD 1

attempt to solve $\frac{{2x}}{{x - 3}} = \frac{{3x}}{{x - 2}}$     (M1)

$2x(x - 2) = 3x(x - 3)$

$x\left[ {2(x - 2) - 3(x - 3)} \right] = 0$

$x(5 - x) = 0$

$x = 0\;\;\;$or$\;\;\;x = 5$     A1A1

METHOD 2

$x = \frac{{3x}}{{x - 2}}\;\;\;$or$\;\;\;x = \frac{{2x}}{{x - 3}}$     (M1)

$x = 0\;\;\;$or$\;\;\;x = 5$     A1A1

[3 marks]

METHOD 1

at ${\text{A}}:\frac{{3x}}{{x - 2}} = \frac{3}{2}$ AND at ${\text{B}}:\frac{{3x}}{{x - 2}} =  - \frac{3}{2}$     M1

$6x = 3x - 6$

$x =  - 2$     A1

$6x = 6 - 3x$

$x = \frac{2}{3}$     A1

solution is $- 2 < x < \frac{2}{3}$     A1

METHOD 2

${\left( {\frac{{3x}}{{x - 2}}} \right)^2} < {\left( {\frac{3}{2}} \right)^2}$     M1

$9{x^2} < \frac{9}{4}{(x - 2)^2}$

$3{x^2} + 4x - 4 < 0$

$(3x - 2)(x + 2) < 0$

$x =  - 2$     (A1)

$x = \frac{2}{3}$     (A1)

solution is $- 2 < x < \frac{2}{3}$     A1

[4 marks]

$- 2 < x < 2$     A1A1

Note:     A1 for correct end points, A1 for correct inequalities.

Note:     If working is shown, then A marks may only be awarded following correct working.

[2 marks]

Total [17 marks]