| Date | November 2015 | Marks available | 6 | Reference code | 15N.2.hl.TZ0.12 |
| Level | HL only | Paper | 2 | Time zone | TZ0 |
| Command term | Explain, Sketch, and State | Question number | 12 | Adapted from | N/A |
Question
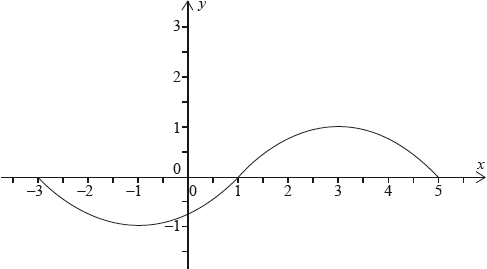
The following graph represents a function y=f(x), where −3≤x≤5.
The function has a maximum at (3, 1) and a minimum at (−1, −1).
The functions u and v are defined as u(x)=x−3, v(x)=2x where x∈R.
(i) State the range of the function u∘f.
(ii) State the range of the function u∘v∘f.
(iii) Find the largest possible domain of the function f∘v∘u.
(i) Explain why f does not have an inverse.
(ii) The domain of f is restricted to define a function g so that it has an inverse g−1.
State the largest possible domain of g.
(iii) Sketch a graph of y=g−1(x), showing clearly the y-intercept and stating the coordinates of the endpoints.
Consider the function defined by h(x)=2x−5x+d, x≠−d and d∈R.
(i) Find an expression for the inverse function h−1(x).
(ii) Find the value of d such that h is a self-inverse function.
For this value of d, there is a function k such that h∘k(x)=2xx+1, x≠−1.
(iii) Find k(x).
Markscheme
Note: For Q12(a) (i) – (iii) and (b) (ii), award A1 for correct endpoints and, if correct, award A1 for a closed interval.
Further, award A1A0 for one correct endpoint and a closed interval.
(i) −4≤y≤−2 A1A1
(ii) −5≤y≤−1 A1A1
(iii) −3≤2x−6≤5 (M1)
Note: Award M1 for f(2x−6).
3≤2x≤11
32≤x≤112 A1A1
[7 marks]
(i) any valid argument eg f is not one to one, f is many to one, fails horizontal line test, not injective R1
(ii) largest domain for the function g(x) to have an inverse is [−1, 3] A1A1
(iii) 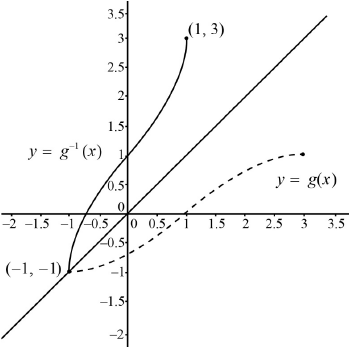
y-intercept indicated (coordinates not required) A1
correct shape A1
coordinates of end points (1, 3) and (−1, −1) A1
Note: Do not award any of the above marks for a graph that is not one to one.
[6 marks]
(i) y=2x−5x+d
(x+d)y=2x−5 M1
Note: Award M1 for attempting to rearrange x and y in a linear expression.
x(y−2)=−dy−5 (A1)
x=−dy−5y−2 (A1)
Note: x and y can be interchanged at any stage
h−1(x)=−dx−5x−2 A1
Note: Award A1 only if h−1(x) is seen.
(ii) self Inverse ⇒h(x)=h−1(x)
2x−5x+d≡−dx−5x−2 (M1)
d=−2 A1
(iii) METHOD 1
2k(x)−5k(x)−2=2xx+1 (M1)
k(x)=x+52 A1
METHOD 2
h−1(2xx+1)=2(2xx+1)−52xx+1−2 (M1)
k(x)=x+52 A1
[8 marks]
Total [21 marks]

