| Date | May 2014 | Marks available | 4 | Reference code | 14M.2.hl.TZ2.7 |
| Level | HL only | Paper | 2 | Time zone | TZ2 |
| Command term | Find | Question number | 7 | Adapted from | N/A |
Question
The function f is defined as f(x)=−3+1x−2, x≠2.
(i) Sketch the graph of y=f(x), clearly indicating any asymptotes and axes intercepts.
(ii) Write down the equations of any asymptotes and the coordinates of any axes intercepts.
Find the inverse function f−1, stating its domain.
Markscheme
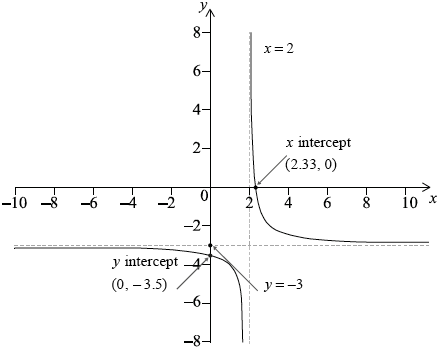 A1A1A1
A1A1A1
Note: Award A1 for correct shape, A1 for x=2 clearly stated and A1 for y=−3 clearly stated.
x intercept (2.33, 0) and y intercept (0, –3.5) A1
Note: Accept –3.5 and 2.33 (7/3) marked on the correct axes.
[4 marks]
x=−3+1y−2 M1
Note: Award M1 for interchanging x and y (can be done at a later stage).
x+3=1y−2
y−2=1x+3 M1
Note: Award M1 for attempting to make y the subject.
f−1(x)=2+1x+3(=2x+7x+3), x≠−3 A1A1
Note: Award A1 only if f−1(x) is seen. Award A1 for the domain.
[4 marks]

