| Date | May 2014 | Marks available | 4 | Reference code | 14M.2.hl.TZ2.13 |
| Level | HL only | Paper | 2 | Time zone | TZ2 |
| Command term | Express, Find, and Hence | Question number | 13 | Adapted from | N/A |
Question
The complex numbers u and v are represented by point A and point B respectively on an Argand diagram.
Point A is rotated through π2 in the anticlockwise direction about the origin O to become point A′. Point B is rotated through π2 in the clockwise direction about O to become point B′.
Consider z=r(cosθ+isinθ), z∈C.
Use mathematical induction to prove that zn=rn(cosnθ+isinnθ), n∈Z+.
Given u=1+√3i and v=1−i,
(i) express u and v in modulus-argument form;
(ii) hence find u3v4.
Plot point A and point B on the Argand diagram.
Find the area of triangle OA′B′.
Given that u and v are roots of the equation z4+bz3+cz2+dz+e=0, where b, c, d, e∈R,
find the values of b, c, d and e.
Markscheme
let P(n) be the proposition zn=rn(cosnθ+isinnθ),n∈¢+
let n=1⇒
LHS=r(cosθ+isinθ)
RHS=r(cosθ+isinθ), ∴P(1) is true R1
assume true for n=k⇒rk(cosθ+isinθ)k=rk(cos(kθ)+isin(kθ)) M1
Note: Only award the M1 if truth is assumed.
now show n=k true implies n=k+1 also true
rk+1(cosθ+isinθ)k+1=rk+1(cosθ+isinθ)k(cosθ+isinθ) M1
=rk+1(cos(kθ)+isin(kθ))(cosθ+isinθ)
=rk+1(cos(kθ)cosθ−sin(kθ)sinθ+i(sin(kθ)cosθ+cos(kθ)sinθ)) A1
=rk+1(cos(kθ+θ)+isin(kθ+θ)) A1
=rk+1(cos(k+1)θ+isin(k+1)θ)⇒n=k+1 is true A1
P(k) true implies P(k+1) true and P(1) is true, therefore by mathematical induction statement is true for n⩾ R1
Note: Only award the final R1 if the first 4 marks have been awarded.
[7 marks]
(i) u = 2{\text{cis}}\left( {\frac{\pi }{3}} \right) A1
v = \sqrt 2 {\text{cis}}\left( { - \frac{\pi }{4}} \right) A1
Notes: Accept 3 sf answers only. Accept equivalent forms.
Accept 2{e^{\frac{\pi }{3}i}} and \sqrt 2 {e^{ - \frac{\pi }{4}i}}.
(ii) {u^3} = {2^3}{\text{cis}}(\pi ) = - 8
{v^4} = 4{\text{cis}}( - \pi ) = - 4 (M1)
{u^3}{v^4} = 32 A1
Notes: Award (M1) for an attempt to find {u^3} and {v^4}.
Accept equivalent forms.
[4 marks]
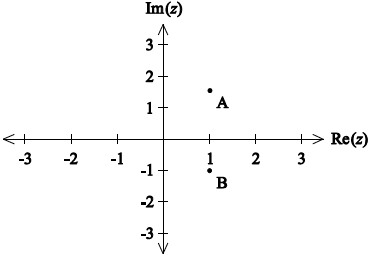 A1
A1
Note: Award A1 if A or {\text{1 + }}\sqrt 3 i and B or 1 - i are in their correct quadrants, are aligned vertically and it is clear that \left| u \right| > \left| v \right|.
[1 mark]
Area = \frac{1}{2} \times 2 \times \sqrt 2 \times \sin \left( {\frac{{5\pi }}{{12}}} \right) M1A1
= 1.37{\text{ }}\left( { = \frac{{\sqrt 2 }}{4}\left( {\sqrt 6 + \sqrt 2 } \right)} \right) A1
Notes: Award M1A0A0 for using \frac{{7\pi }}{{12}}.
[3 marks]
(z - 1 + {\text{i}})(z - 1 - {\text{i}}) = {z^2} - 2z + 2 M1A1
Note: Award M1 for recognition that a complex conjugate is also a root.
\left( {z - 1 - \sqrt 3 {\text{i}}} \right)\left( {z - 1 + \sqrt 3 {\text{i}}} \right) = {z^2} - 2z + 4 A1
\left( {{z^2} - 2z + 2} \right)\left( {{z^2} - 2z + 4} \right) = {z^4} - 4{z^3} + 10{z^2} - 12z + 8 M1A1
Note: Award M1 for an attempt to expand two quadratics.
[5 marks]

