| Date | May 2018 | Marks available | 1 | Reference code | 18M.2.SL.TZ1.6 |
| Level | Standard level | Paper | Paper 2 | Time zone | Time zone 1 |
| Command term | Identify | Question number | 6 | Adapted from | N/A |
Question
The radioactive nuclide beryllium-10 (Be-10) undergoes beta minus (β–) decay to form a stable boron (B) nuclide.
The initial number of nuclei in a pure sample of beryllium-10 is N0. The graph shows how the number of remaining beryllium nuclei in the sample varies with time.
An ice sample is moved to a laboratory for analysis. The temperature of the sample is –20 °C.
Identify the missing information for this decay.
On the graph, sketch how the number of boron nuclei in the sample varies with time.
After 4.3 × 106 years,
\[\frac{{{\text{number of produced boron nuclei}}}}{{{\text{number of remaining beryllium nuclei}}}} = 7.\]
Show that the half-life of beryllium-10 is 1.4 × 106 years.
Beryllium-10 is used to investigate ice samples from Antarctica. A sample of ice initially contains 7.6 × 1011 atoms of beryllium-10. State the number of remaining beryllium-10 nuclei in the sample after 2.8 × 106 years.
State what is meant by thermal radiation.
Discuss how the frequency of the radiation emitted by a black body can be used to estimate the temperature of the body.
Calculate the peak wavelength in the intensity of the radiation emitted by the ice sample.
Derive the units of intensity in terms of fundamental SI units.
Markscheme
\(_{{\mkern 1mu} {\mkern 1mu} 4}^{10}{\text{Be}} \to _{{\mkern 1mu} {\mkern 1mu} 5}^{10}{\text{B}} + \beta + {\overline {\text{V}} _{\text{e}}}\)
conservation of mass number AND charge \(_{\,\,5}^{10}{\text{B}}\), \(_{{\mkern 1mu} {\mkern 1mu} 4}^{10}{\text{Be}}\)
Correct identification of both missing values required for [1].
[1 mark]
correct shape ie increasing from 0 to about 0.80 N0
crosses given line at 0.50 N0
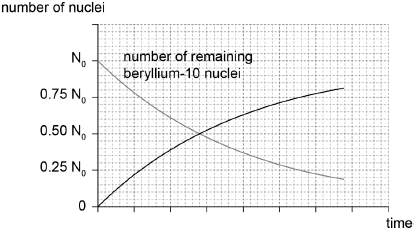
[2 marks]
ALTERNATIVE 1
fraction of Be = \(\frac{1}{8}\), 12.5%, or 0.125
therefore 3 half lives have elapsed
\({t_{\frac{1}{2}}} = \frac{{4.3 \times {{10}^6}}}{3} = 1.43 \times {10^6}\) «≈ 1.4 × 106» «y»
ALTERNATIVE 2
fraction of Be = \(\frac{1}{8}\), 12.5%, or 0.125
\(\frac{1}{8} = {{\text{e}}^{ - \lambda }}(4.3 \times {10^6})\) leading to λ = 4.836 × 10–7 «y»–1
\(\frac{{\ln 2}}{\lambda }\) = 1.43 × 106 «y»
Must see at least one extra sig fig in final answer.
[3 marks]
1.9 × 1011
[1 mark]
emission of (infrared) electromagnetic/infrared energy/waves/radiation.
[1 mark]
the (peak) wavelength of emitted em waves depends on temperature of emitter/reference to Wein’s Law
so frequency/color depends on temperature
[2 marks]
\(\lambda = \frac{{2.90 \times {{10}^{ - 3}}}}{{253}}\)
= 1.1 × 10–5 «m»
Allow ECF from MP1 (incorrect temperature).
[2 marks]
correct units for Intensity (allow W, Nms–1 OR Js–1 in numerator)
rearrangement into proper SI units = kgs–3
Allow ECF for MP2 if final answer is in fundamental units.
[2 marks]

