| Date | May 2012 | Marks available | 6 | Reference code | 12M.2.hl.TZ2.9 |
| Level | HL | Paper | 2 | Time zone | TZ2 |
| Command term | Calculate and Identify | Question number | 9 | Adapted from | N/A |
Question
The reaction between carbon monoxide, CO(g), and nitrogen dioxide, NO2(g), was studied at different temperatures and a graph was plotted of lnk against 1T. The equation of the line of best fit was found to be:
lnk=−1.60×104(1T)+23.2
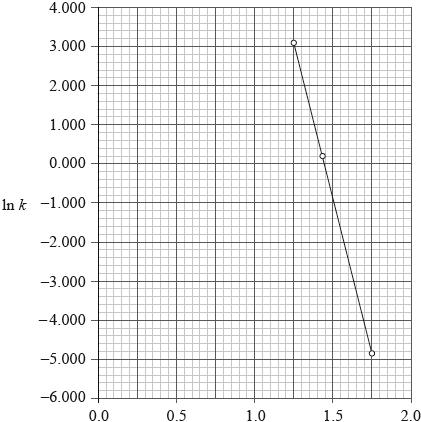
1T/10−3K−1
(i) State the full electron configuration of Fe.
(ii) State the abbreviated electron configuration of Fe3+ ions.
(iii) Cyanide ions, CN−, can act as ligands. One complex ion that involves the cyanide ion is [Fe(CN)6]3−. Identify the property of a cyanide ion which allows it to act as a ligand, and explain the bonding that occurs in the complex ion in terms of acid–base theory. Describe the structure of the complex ion, [Fe(CN)6]3−.
(iv) Explain why complexes of Fe3+ are coloured.
(i) The Arrhenius equation is shown in Table 1 of the Data Booklet. Identify the symbols k and A.
k:
A:
(ii) Calculate the activation energy, Ea, for the reaction between CO(g) and NO2(g).
(iii) Calculate the numerical value of A.
Markscheme
(i) 1s22s22p63s23p63d64s2/1s22s22p63s23p64s23d6;
(ii) [Ar]3d5;
(iii) lone pair of electrons (on C);
CN− acts as a Lewis base / Fe3+ acts as a Lewis acid;
dative covalent/coordinate bond formed (between CN− and Fe3+);
ligands occupy an octahedral shape around central metal ion / coordination number of Fe3+ is 6;
(iv) d sub-level splits (into two sets of orbitals of different energy) /  ΔE;
ΔE;
colour due to electron transitions between (split) d orbitals;
(i) k:
rate constant;
A:
Arrhenius constant / frequency/pre-exponential factor;
(ii) gradient=−EaR/Ea=−gradient×R;
(=−(−16)×8.31)=+133 (kJmol−1)/1.33×105 (Jmol−1);
(iii) lnA=(intercept on y - axis=) 23.2;
A=1.190×1010;
Examiners report
Most candidates had no difficulty with the full electron configuration of Fe in (a) but many could not write the abbreviated electron configuration of Fe3+, losing 3d electrons ahead of 4s. Some G2 comments suggested that the word abbreviated caused problems, but this is stated in the teacher‘s notes and most candidates seemed to have no difficulty with the term. Descriptions of cyanide ions acting as ligands were particularly well expressed but some candidates had difficulty explaining why Fe3+ ions are coloured, referring to excited orbitals emitting light.
In part (c) most candidates identified the symbols of the Arrhenius equation. Many calculated the activation energy, although several calculated the gradient from the graph rather than using the equation of the line of best fit. (This was accepted, but made the question much harder than intended.) Several candidates also calculated the numerical value of A correctly.

