| Date | May 2015 | Marks available | 3 | Reference code | 15M.2.sl.TZ2.6 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Estimate | Question number | 6 | Adapted from | N/A |
Question
The following table shows the number of bicycles, x, produced daily by a factory and their total production cost, y, in US dollars (USD). The table shows data recorded over seven days.
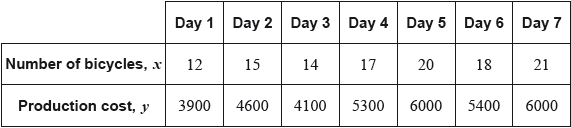
(i) Write down the Pearson’s product–moment correlation coefficient, r, for these data.
(ii) Hence comment on the result.
Write down the equation of the regression line y on x for these data, in the form y=ax+b.
Estimate the total cost, to the nearest USD, of producing 13 bicycles on a particular day.
All the bicycles that are produced are sold. The bicycles are sold for 304 USD each.
Explain why the factory does not make a profit when producing 13 bicycles on a particular day.
All the bicycles that are produced are sold. The bicycles are sold for 304 USD each.
(i) Write down an expression for the total selling price of x bicycles.
(ii) Write down an expression for the profit the factory makes when producing x bicycles on a particular day.
(iii) Find the least number of bicycles that the factory should produce, on a particular day, in order to make a profit.
Markscheme
(i) r=0.985(0.984905…) (G2)
Notes: If unrounded answer is not seen, award (G1)(G0) for 0.99 or 0.984. Award (G2) for 0.98.
(ii) strong, positive (A1)(A1)
y=259.909…x+698.648…(y=260x+699) (G1)(G1)
Notes: Award (G1) for 260x and (G1) for 699. If the answer is not an equation award a maximum of (G1)(G0).
y=259.909…×13+698.648… (M1)
Note: Award (M1) for substitution of 13 into their regression line equation from part (b).
y=4077.47… (A1)(ft)(G2)
y=4077 (USD) (A1)(ft)
Notes: Follow through from their answer to part (b). If rounded values from part (b) used, answer is 4079. Award the final (A1)(ft) for a correct rounding to the nearest USD of their answer. The unrounded answer may not be seen.
If answer is 4077 and no working is seen, award (G2).
13×304−(4077.47)=−125.477…(−125)OR
4077.47−(13×304)=125.477…(125) (M1)
Notes: Award (M1) for calculating the difference between 13×304 and their answer to part (c).
If rounded values are used in equation, answer is −127.
profit is negativeORcost>sales (A1)
OR
13×304=3952 (M1)
Note: Award (M1) for calculating the price of 13 bikes.
3952<4077.47 (A1)(ft)
Note: Award (A1) for showing 3952 is less than their part (c). This may be communicated in words. Follow through from part (c), but only if value is greater than 3952.
OR
407713=313.62 (M1)
Note: Award (M1) for calculating the cost of 1 bicycle.
313.62>304 (A1)(ft)
Note: Award (A1) for showing 313.62 is greater than 304. This may be communicated in words. Follow through from part (c), but only if value is greater than 304.
OR
4077304=13.41 (M1)
Note: Award (M1) for calculating the number of bicycles that should have been be sold to cover total cost.
13.41>13 (A1)(ft)
Note: Award (A1) for showing 13.41 is greater than 13. This may be communicated in words. Follow through from part (c), but only if value is greater than 13.
(i) 304x (A1)
(ii) 304x−(259.909…x+698.648…) (A1)(ft)(A1)(ft)
Note: Award (A1)(ft) for difference between their answers to parts (b) and (e)(i), (A1)(ft) for correct expression.
(iii) 304x−(259.909…x+698.648…)>0 (M1)
Notes: Award (M1) for comparing their expression in part (e)(ii) to 0. Accept an equation. Accept 3040x−y>0 or equivalent.
x=16 bicycles (A1)(ft)(G2)
Notes: Follow through from their answer to part (b). Answer must be a positive integer greater than 13 for the (A1)(ft) to be awarded.
Award (G1) for an answer of 15.84.

