| Date | May 2010 | Marks available | 4 | Reference code | 10M.2.sl.TZ1.3 |
| Level | SL only | Paper | 2 | Time zone | TZ1 |
| Command term | Sketch | Question number | 3 | Adapted from | N/A |
Question
Let f(x)=xcosx , for 0≤x≤6 .
Find f′(x) .
[3]
a.
On the grid below, sketch the graph of y=f′(x) .
[4]
b.
Markscheme
evidence of choosing the product rule (M1)
e.g. x×(−sinx)+1×cosx
f′(x)=cosx−xsinx A1A1 N3
[3 marks]
a.
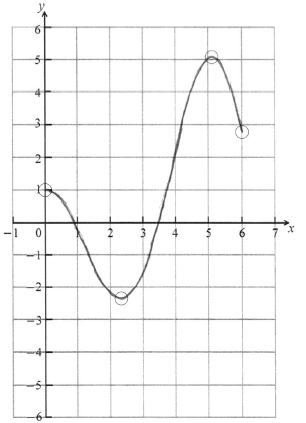A1A1A1A1 N4
Note: Award A1 for correct domain, 0≤x≤6 with endpoints in circles, A1 for approximately correct shape, A1 for local minimum in circle, A1 for local maximum in circle.
[4 marks]
b.
Examiners report
This problem was well done by most candidates. There were some candidates that struggled to apply the product rule in part (a) and often wrote nonsense like −xsinx=−sinx2 .
a.
In part (b), few candidates were able to sketch the function within the required domain and a large number of candidates did not have their calculator in the correct mode.
b.
Syllabus sections
Topic 2 - Functions and equations » 2.2 » Use of technology to graph a variety of functions, including ones not specifically mentioned.
Show 27 related questions
