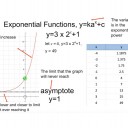
 y = kax+c
y = kax+c
The exponential function is set apart from other families of functions because of the fundamental impact of having the variable in the exponent. It stops being a multiplier and starts being more 'powerful' (forgive the bad joke). These functions can be counterintuitive as the speed with which their value increases can become extraordinary. One professor famously said that 'The biggest single failing of the human race is our inability to understand the exponential function. There is also a strong link here with Geometric sequences.
In this unit you should learn to…
-
recognise exponential functions
-
find the y-intercepts of exponential functions
-
find the asymptotes of exponential functions
This section of the page can be used for quick review. The flashcards help you go over key points and the quiz lets you practice answering questions on this subtopic.
Flash Card
Review this condensed 'key point' flashcard to help you check and keep ideas fresh in your mind.
Self Checking Quiz
Practice your understanding on these quiz questions. Check your answers when you are done and read the hints where you got stuck. If you find there are still some gaps in your understanding then go back to the videos and slides above.
.....
How much of 2.5 Exponential Models have you understood?













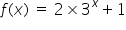 ,
,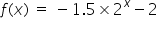 ,
,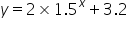
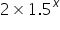 can never be zero, y can never be 3.2
can never be zero, y can never be 3.2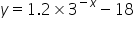
 can never be zero, y can never be -18
can never be zero, y can never be -18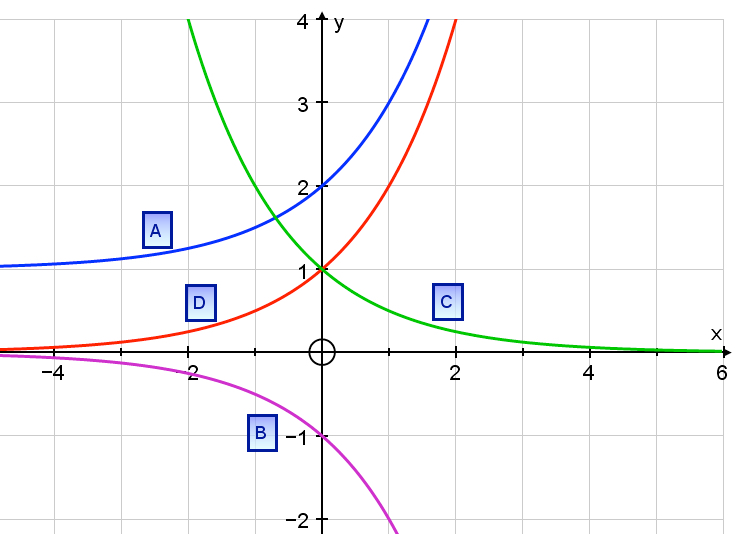
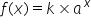 , what are the values of k and a?
, what are the values of k and a? , which measures temperature (T) as time (x, in minutes) passes.
, which measures temperature (T) as time (x, in minutes) passes. 
.jpg)

 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn