On this page you can find examination questions from the topic of functions


The line \(l_1\) has equation 2x+5y+6=0
The line \(l_2\) is perpendicular to the line \(l_1\)and passes through the point (2 , -2)
a) Find the equation of \(l_2\)in the form ax+by+d=0, where a, b and d are constants.
b) Find the coordinates where \(l_2\) meets the y axis
Hint
Full Solution


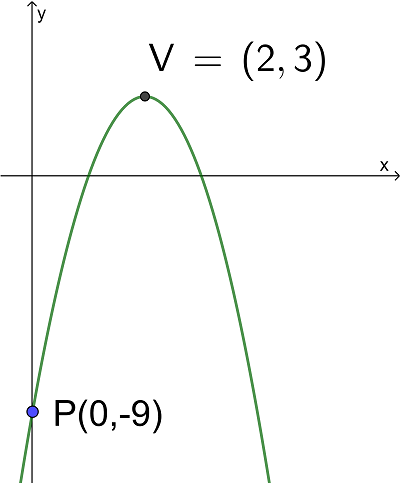
a) The following diagram shows the graph of a function f
On the same set of axes, sketch the graph of f(-x) + 2
You can print this graph from here

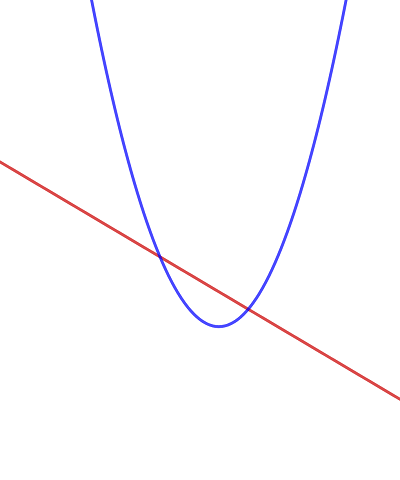
b)
The following diagram shows the function af(x+b)
Write down the values of a and b

Hint
Full Solution
How much of Functions Examination Questions SL have you understood?








 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn