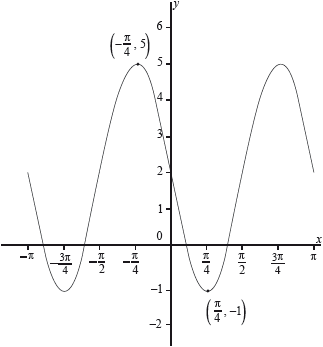
Find the value of

(i)     \(A\);

(ii)     \(B\);

(iii)     \(C\).

Solve \(f(x) = 3\) for \(0 \le x \le \pi \).

(i)     \(A =  - 3\)     A1

(ii)     period \( = \frac{\pi }{B}\)     (M1)

\(B = 2\)     A1

Note:     Award as above for \(A = 3\) and \(B =  - 2\).

(iii)     \(C = 2\)     A1

[4 marks]

\(x = 1.74,{\text{ }}2.97\;\;\;\left( {x = \frac{1}{2}\left( {\pi  + \arcsin \frac{1}{3}} \right),{\text{ }}\frac{1}{2}\left( {2\pi  - \arcsin \frac{1}{3}} \right)} \right)\)     (M1)A1

Note:     Award (M1)A0 if extra correct solutions eg \(( - 1.40,{\text{ }} - 0.170)\) are given outside the domain \(0 \le x \le \pi \). Do not award FT in (b).

[2 marks]

Total [6 marks]