| Date | November 2017 | Marks available | 2 | Reference code | 17N.2.hl.TZ0.11 |
| Level | HL only | Paper | 2 | Time zone | TZ0 |
| Command term | Show that and Hence | Question number | 11 | Adapted from | N/A |
Question
Consider the function f(x)=2sin2x+7sin2x+tanx−9, 0⩽x<π2.
Let u=tanx.
Determine an expression for f′(x) in terms of x.
Sketch a graph of y=f′(x) for 0⩽x<π2.
Find the x-coordinate(s) of the point(s) of inflexion of the graph of y=f(x), labelling these clearly on the graph of y=f′(x).
Express sinx in terms of μ.
Express sin2x in terms of u.
Hence show that f(x)=0 can be expressed as u3−7u2+15u−9=0.
Solve the equation f(x)=0, giving your answers in the form arctank where k∈Z.
Markscheme
f′(x)=4sinxcosx+14cos2x+sec2x (or equivalent) (M1)A1
[2 marks]
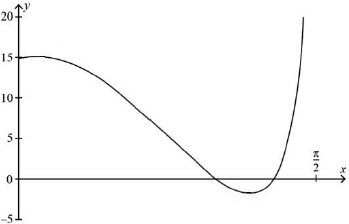 A1A1A1A1
A1A1A1A1
Note: Award A1 for correct behaviour at x=0, A1 for correct domain and correct behaviour for x→π2, A1 for two clear intersections with x-axis and minimum point, A1 for clear maximum point.
[4 marks]
x=0.0736 A1
x=1.13 A1
[2 marks]
attempt to write sinx in terms of u only (M1)
sinx=u√1+u2 A1
[2 marks]
cosx=1√1+u2 (A1)
attempt to use sin2x=2sinxcosx (=2u√1+u21√1+u2) (M1)
sin2x=2u1+u2 A1
[3 marks]
2sin2x+7sin2x+tanx−9=0
2u21+u2+14u1+u2+u−9 (=0) M1
2u2+14u+u(1+u2)−9(1+u2)1+u2=0 (or equivalent) A1
u3−7u2+15u−9=0 AG
[2 marks]
u=1 or u=3 (M1)
x=arctan(1) A1
x=arctan(3) A1
Note: Only accept answers given the required form.
[3 marks]

