Show that the opposite angles of a cyclic quadrilateral add up to ${180^ \circ }$ .

A quadrilateral ABCD is inscribed in a circle $S$ . The four tangents to $S$ at the vertices A, B, C and D form the edges of a quadrilateral EFGH. Given that EFGH is cyclic, show that AC and BD intersect at right angles.

Show that the locus of a point ${\rm{P'}}$ , which satisfies $\overrightarrow {{\rm{QP'}}}  = k\overrightarrow {{\rm{QP}}}$ , is a circle $C'$ , where k is a constant and $0 < k < 1$ .

Show that the two tangents to $C$ from Q are also tangents to ${\rm{C'}}$ .

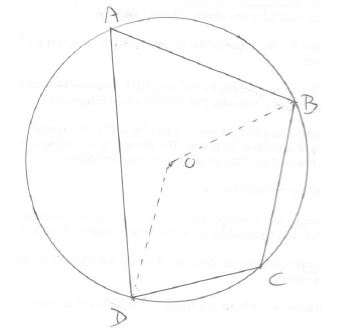
recognition of relevant theorem     (M1)

eg ${\rm{D}}\hat {\rm{O}}{\rm{B}} = 2 \times {\rm{D}}\hat {\rm{A}}{\rm{B}}$     A1

${360^ \circ } - {\rm{D}}\hat {\rm{O}}{\rm{B}} = 2 \times {\rm{D}}\hat {\rm{C}}{\rm{B}}$     A1

so ${\rm{D}}\hat {\rm{A}}{\rm{B}} + {\rm{D}}\hat {\rm{C}}{\rm{B}} = {\rm{18}}{0^ \circ }$     AG

[3 marks]

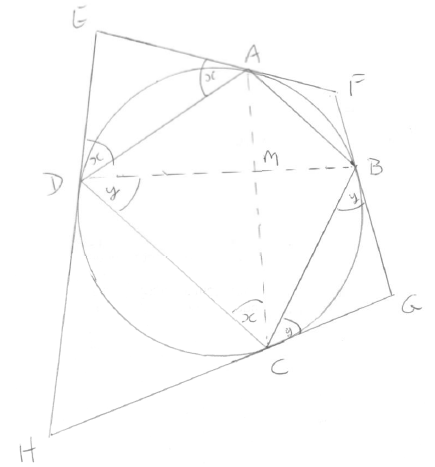
diagram showing tangents EAF, FBG, GCH and HDE; diagonals cross at M.     M1

let $x = {\rm{E}}\hat {\rm{D}}{\rm{A}} = {\rm{E}}\hat {\rm{A}}{\rm{D}}$ ; $y = {\rm{B}}\hat {\rm{C}}{\rm{G}} = {\rm{C}}\hat {\rm{B}}{\rm{G}}$     A1

${\rm{D}}\hat {\rm{E}}{\rm{A}} + {\rm{H}}\hat {\rm{G}}{\rm{F}} = 180 - 2x + 180 - 2y = 360 - 2(x + y)$     M1A1

${\rm{C}}\hat {\rm{D}}{\rm{B}} = y$ and ${\rm{A}}\hat {\rm{C}}{\rm{D}} = x$ , as angles in alternate segments     M1A1

${\rm{D}}\hat {\rm{M}}{\rm{C}} = 180 - (x + y) = \left( {\frac{1}{2}} \right)({\rm{D}}\hat {\rm{E}}{\rm{A}} + {\rm{H}}\hat {\rm{G}}{\rm{F}}) = {90^ \circ }$     A1

so the diagonals cross at right angles     AG

[7 marks]

                                                                     M1

let ${\rm{O'}}$ be the point on OQ such ${\rm{O'P'}}$ is parallel to OP     A1

using similar triangles ${\rm{O'Q}} = k{\rm{OQ}}$ , so ${\rm{O'}}$ is a fixed point     M1A1

and ${\rm{O'P}} = k{\rm{OP}}$ which is constant     A1

so ${\rm{P'}}$ lies on a circle centre ${\rm{O'}}$     R1

so the locus of ${\rm{P'}}$ is a circle     AG

[6 marks]

let one of the two tangents to $C$ from Q touch $C$ at T

the image of T lies on TQ     A1

and is a unique point ${{\rm{T'}}}$ on ${C'}$     A1

so ${\rm{TT'}}$ is a common tangent and passes through Q     R1

the same is true for the other tangent     A1

so the two tangents to $C$ from Q are also tangents to ${C'}$     AG

[4 marks]

(a) Most candidates produced a valid answer, although a small minority used a circular argument.

(b) A few candidates went straight to the core of this question. However, many other candidates produced incoherent answers containing some true statements, some irrelevancies and some incorrect statements, based on a messy diagram.

(a) This was poorly answered. Many candidates failed to note that the points Q, P and its image were defined to be collinear, and tried to invoke the notion of the Apollonius Circle theory. Others tried a coordinate approach – in principle this could work, but is actually quite tricky without a sensible choice of axes and the origin.