| Date | May 2010 | Marks available | 2 | Reference code | 10M.1.hl.TZ0.6 |
| Level | HL only | Paper | 1 | Time zone | TZ0 |
| Command term | Show that | Question number | 6 | Adapted from | N/A |
Question
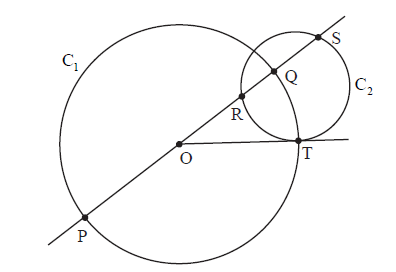
The figure shows a circle C1 with centre O and diameter [PQ] and a circle C2 which intersects (PQ) at the points R and S. T is one point of intersection of the two circles and (OT) is a tangent to C2 .
Show that OROT=OTOS .
(i) Show that PR−RQ=2OR .
(ii) Show that PR−RQPR+RQ=PS−SQPS+SQ .
Markscheme
by the tangent – secant theorem, M1
OT2=OR∙OS A1
so that OROT=OTOS AG
[2 marks]
(i) PR−RQ=PO+OR−(OQ−OR) A1
=2OR AG
(ii) attempt to continue the process set up in (b)(i) (M1)
PR+RQ=PO+OR+OQ−OR=2OT A1
PS−SQ=PQ+QS−SQ=2OT A1
PS+SQ=PO+OS−OQ=2OS A1
it now follows that
PR−RQPR+RQ=OROT and PS−SQPS+SQ=OTOS so using the result in part (a) R1
PR−RQPR+RQ=PS−SQPS+SQ AG
[6 marks]
Examiners report
Most candidates solved (a) correctly although some used similar triangles instead of the more obvious tangent-secant theorem.
Although (b) and then (c) were fairly well signposted, many candidates were unable to cope with the required algebra.

