${\text{ABCDEF}}$ is a hexagon. A circle lies inside the hexagon and touches each of the six sides.

Show that ${\text{AB}} + {\text{CD}} + {\text{EF}} = {\text{BC}} + {\text{DE}} + {\text{FA}}$.

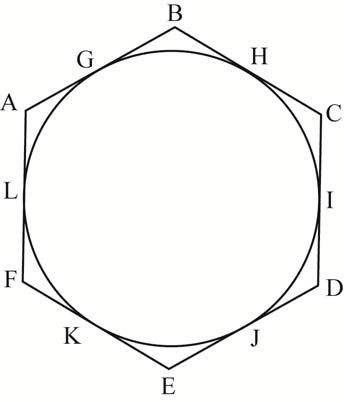     A1

the lengths of the two tangents from a point to a circle are equal     (R1)

so that

${\text{AG}} = {\text{LA}}$

${\text{GB}} = {\text{BH}}$

${\text{CI}} = {\text{HC}}$

${\text{ID}} = {\text{DJ}}$

${\text{EK}} = {\text{JE}}$

${\text{KF}} = {\text{ FL}}$     A1

adding,

${\text{(AG}} + {\text{GB)}} + {\text{(CI}} + {\text{ID)}} + {\text{(EK}} + {\text{KF)}} = {\text{(BH}} + {\text{HC)}} + {\text{(DJ}} + {\text{JE)}} + {\text{(FL}} + {\text{LA)}}$     M1A1

${\text{AB}} + {\text{CD}} + {\text{EF}} = {\text{BC}} + {\text{DE}} + {\text{FA}}$     AG

[5 marks]