| Date | November 2014 | Marks available | 1 | Reference code | 14N.2.sl.TZ0.6 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Write down | Question number | 6 | Adapted from | N/A |
Question
The following diagram shows two triangles, OBC and OBA, on a set of axes. Point C lies on the y-axis, and O is the origin.
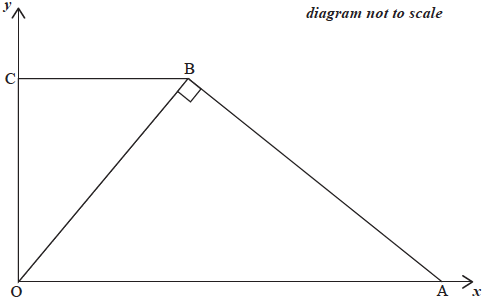
The equation of the line BC is y=4.
Write down the coordinates of point C.
The x-coordinate of point B is a.
(i) Write down the coordinates of point B;
(ii) Write down the gradient of the line OB.
Point A lies on the x-axis and the line AB is perpendicular to line OB.
(i) Write down the gradient of line AB.
(ii) Show that the equation of the line AB is 4y+ax−a2−16=0.
The area of triangle AOB is three times the area of triangle OBC.
Find an expression, in terms of a, for
(i) the area of triangle OBC;
(ii) the x-coordinate of point A.
Calculate the value of a.
Markscheme
(0, 4) (A1)
Notes: Accept x=0, y=4.
(i) (a, 4) (A1)(ft)
Notes: Follow through from part (a).
(ii) 4a (A1)(ft)
Note: Follow through from part (b)(i).
(i) −a4 (A1)(ft)
Note: Follow through from part (b)(ii).
(ii) y=−a4x+c (M1)
Note: Award (M1) for substitution of their gradient from part (c)(i) in the equation.
4=−a4×a+c
c=14×a2+4
y=−a4x+14a2+4 (A1)
OR
y−4=−a4(x−a) (M1)
Note: Award (M1) for substitution of their gradient from part (c)(i) in the equation.
y=−ax4+a24+4 (A1)
4y=−ax+a2+16
4y+ax−a2−16=0 (AG)
Note: Both the simplified and the not simplified equations must be seen for the final (A1) to be awarded.
(i) 2a (A1)
(ii) 4x2=3×2a (M1)
Note: Award (M1) for correct equation.
x=3a (A1)(ft)
Note: Follow through from part (d)(i).
OR
0−4=−a4(x−a) (M1)
Note: Award (M1) for correct substitution of their gradient and the coordinates of their point into the equation of a line.
16a=x−a
x=a+16a (A1)(ft)
Note: Follow through from parts (b)(i) and (c)(i).
OR
4×0+ax−a2−16=0 (M1)
Note: Award (M1) for correct substitution of the coordinates of A(x, 0) into the equation of line AB.
ax−a2−16=0
x=a+16aORx=(a2+16)a (A1)(G1)
4(0)+a(3a)−a2−16=0 (M1)
Note: Award (M1) for correct substitution of their 3a from part (d)(ii) into the equation of line AB.
OR
12(a+16a)×4=3(4a2) (M1)
Note: Award (M1) for area of triangle AOB (with their substituted a+16a and 4) equated to three times their area of triangle AOB.
a=2.83(2.82842..., 2√2, √8) (A1)(ft)(G1)
Note: Follow through from parts (d)(i) and (d)(ii).

