| Date | May 2014 | Marks available | 4 | Reference code | 14M.1.sl.TZ2.7 |
| Level | SL only | Paper | 1 | Time zone | TZ2 |
| Command term | Determine | Question number | 7 | Adapted from | N/A |
Question
The diagram shows a wheelchair ramp, A, designed to descend from a height of 80 cm.
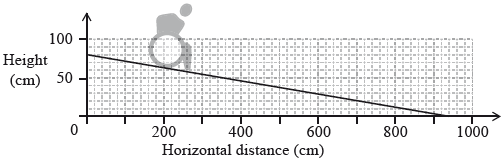
Use the diagram above to calculate the gradient of the ramp.
The gradient for a safe descending wheelchair ramp is −112.
Using your answer to part (a), comment on why wheelchair ramp A is not safe.
The equation of a second wheelchair ramp, B, is 2x+24y−1920=0.
(i) Determine whether wheelchair ramp B is safe or not. Justify your answer.
(ii) Find the horizontal distance of wheelchair ramp B.
Markscheme
−80940 (−0.0851,−0.085106…,−447) (A1) (C1)
[1 mark]
−0.0851 (−0.085106…)<−112(−0.083333…) (A1)(ft) (C1)
Notes: Accept “less than” in place of inequality.
Award (A0) if incorrect inequality seen.
Follow through from part (a).
[1 mark]
(i) ramp B is safe (A1)
the gradient of ramp B is −112 (R1)
Notes: Award (R1) for “the gradient of ramp B is −112” seen.
Do not award (A1)(R0).
(ii) 2x=1920 (M1)
Note: Accept alternative methods.
960 (cm) (A1) (C4)
[4 marks]

