| Date | May 2008 | Marks available | 4 | Reference code | 08M.1.sl.TZ2.6 |
| Level | SL only | Paper | 1 | Time zone | TZ2 |
| Command term | Calculate | Question number | 6 | Adapted from | N/A |
Question
There are 20 students in a classroom. Each student plays only one sport. The table below gives their sport and gender.
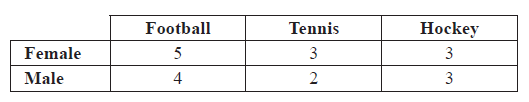
One student is selected at random.
(i) Calculate the probability that the student is a male or is a tennis player.
(ii) Given that the student selected is female, calculate the probability that the student does not play football.
Two students are selected at random. Calculate the probability that neither student plays football.
Markscheme
(i) correct calculation (A1)
e.g. \(\frac{9}{{20}} + \frac{5}{{20}} - \frac{2}{{20}}\) , \(\frac{{4 + 2 + 3 + 3}}{{20}}\)
\({\text{P(male or tennis)}} = \frac{{12}}{{20}}\) A1 N2
(ii) correct calculation (A1)
e.g. \(\frac{6}{{20}} \div \frac{{11}}{{20}}\) , \(\frac{{3 + 3}}{{11}}\)
\({\text{P(not football|female)}} = \frac{6}{{11}}\) A1 N2
[4 marks]
\({\text{P(first not football)}} = \frac{{11}}{{20}}\) , \({\text{P(second not football)}} = \frac{{10}}{{19}}\) A1
\({\text{P(neither football)}} = \frac{{11}}{{20}} \times \frac{{10}}{{19}}\) A1
\({\text{P(neither football)}} = \frac{{110}}{{380}}\) A1 N1
[3 marks]
Examiners report
Many candidates had difficulty with this question, usually as a result of seeking to solve the problem by formula instead of looking carefully at the table frequencies.
A very common error in part (b) was to assume identical probabilities for each selection instead of dependent probabilities where there is no replacement.
