In the early 11^th century the great Islamic scientist al-Biruni (born 973, died 1048 - full name Abu Arrayhan Muhammad ibn Ahmad al-Biruni) devised a method to efficiently measure the radius of the earth. A little more than a thousand years earlier, the Greek mathematician Eratosthenes (276-194 BC) had computed the circumference of the earth but his method required measuring a distance between two cities far apart from each other by ordering soldiers to walk the distance and count their steps. Al-Biruni’s method could be performed by a single person measuring three angles and a much shorter distance. But, the most important aspect of al-Biruni’s clever method was the application of some algebra and trigonometry which allowed him to derive two important formulas.

Your task is to derive the two formulas and then given al-Biruni’s measurements compute his value for the radius of the earth – and also the earth’s circumference.

A secondary task involves considering how much al-Biruni’s method (Eratosthenes’ method too) relied on very accurate angle measurements.

1. Find a formula for the height, h, of a mountain in terms of two angles of elevation, ${\text{θ}}_1$ and ${\text{θ}}_2$ , and the distance, d, between the points where ${\text{θ}}_1$ and ${\text{θ}}_2$ were measured from.

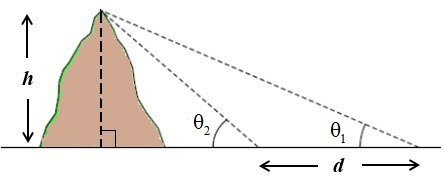
2. Find a formula for the radius of the earth, R, in terms of the angle of depression, $\text{φ}$ .

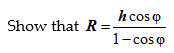
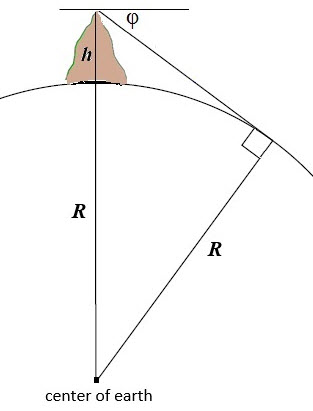
3. For ${\text{θ}}_1$ and ${\text{θ}}_2$ , al-Biruni measured angles of ${5.5}^{\circ }$ and ${7.5}^{\circ }$ respectively. The distance d between the points where ${\text{θ}}_1$ and ${\text{θ}}_2$ were measured was 875.11 meters. The angle of depression, $\text{φ}$ , measured at the top of the mountain to the horizon was ${0.57}^{\circ }$ . Using the two formulas from 1 and 2, calculate the radius and circumference of the earth in kilometers accurate to four significant figures.

4. If the angle of depression, $\text{φ}$ , measured from the top of the mountain to the horizon was off by one tenth of a degree then this would be a percentage error of approximately 17.5% (0.10 is about 17.5% of 0.57). (a) If $\text{φ=0}{\text{.47}}^{\circ }$ then by what percent would it change the value of the earth’s radius obtained above for $\text{φ=0}{\text{.57}}^{\circ }$ ? (b) If $\text{φ=0}{\text{.67}}^{\circ }$ then by what percent would it change the value of the earth’s radius obtained above for $\text{φ=0}{\text{.57}}^{\circ }$ ?

download document with all the above text and figures - along with answers to parts 3 & 4 on last page

Radius of the Earth