 Torque, also known as the moment of a force, is defined as the product of a force and the perpendicular distance from the line of action to the pivot.
Torque, also known as the moment of a force, is defined as the product of a force and the perpendicular distance from the line of action to the pivot.
Key Concepts
Torque is the rotational equivalent of force.
What if the force isn't perpendicular to th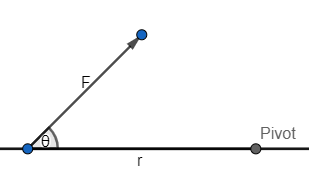 e beam?
e beam?
\(\Gamma=Fr\sin \theta\)
- \(\Gamma\) is torque (Nm)
- \(F\) is the force acting (N)
- \(r\) is the distance between the line of action of the force and the pivot
- \(\theta\) is the angle between the force direction and the beam
A couple is a body with two equal and opposite forces acting on it, at opposite ends. These forces combine to increase the torque on the body.
couple is a body with two equal and opposite forces acting on it, at opposite ends. These forces combine to increase the torque on the body.
In this case, the total torque acting is:
\(\Gamma=Fr\sin 90+Fr\sin 90\)
\(\Gamma=2Fr\)

Moment of inertia is the rotational equivalent of mass. The moment of inertia of a rigid body is defined as the sum of the products of the mass and the squares of their distance from the pivot of all the particles in the body.
\(I=\sum mr^2\)
- \(I\) is moment of intertia (kgm2)
- \(m\) is the mass of a discrete section of the body (kg)
- \(r\) is the distance between the centre of mass of the discrete section and the pivot (m)
The moment of inertia of some bodies is easy to calculate. You will have to do this for yourself in the exam.
For 3 point masses orbitting a common pivot on massless rods, the moment of inertia is the sum of the moment of inertia of the objects: \(I =M_1{r_1}^2+M_2{r_2}^2+M_3{r_3}^2\)

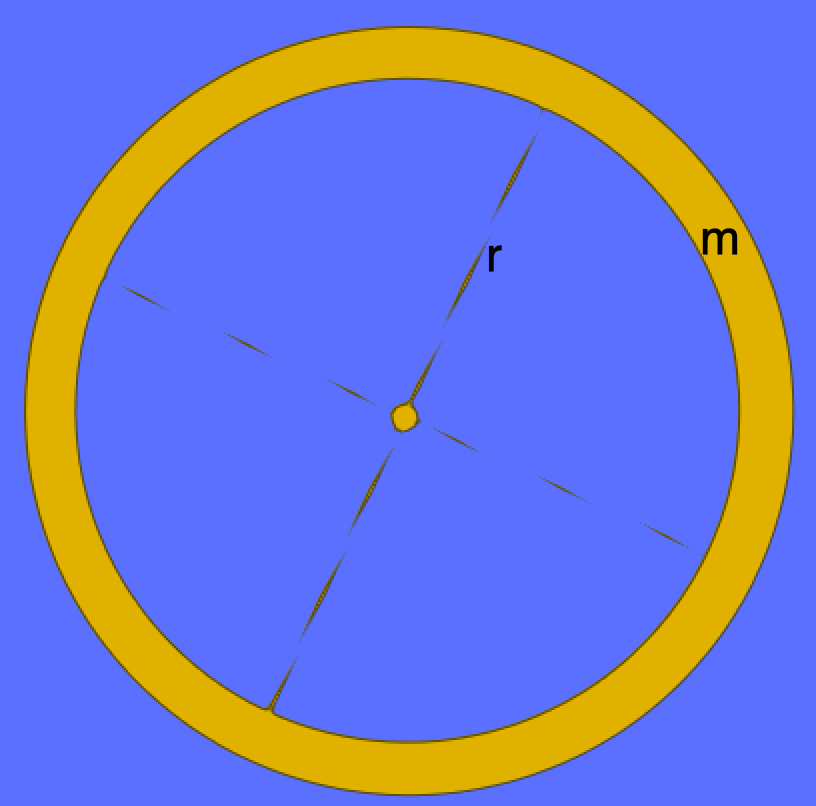
For a loop rotating around its centre, all particles can be assumed to be a constant distance from the pivot and so the total mass is at the radius: \(I =mr^2\)
The moment of inertia of more complex 3-dimensional shapes is harder to calculate and involves challenging integration from first principles. You will be given the equation for the moment of inertia in the exam. Note that there may be several different moment of intertia values for the same object, depending on the location of the pivot.
How much of Torque and moment of inertia have you understood?











 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn