 Don't be fooled into thinking that only solids are 'heavy' and that we can approximate everything as a volume-less particle. Fluids, such as liquids, have a density, exert an upward buoyancy on anything that should displace them, have increasing pressure with depth and are used in hydraulics to exert huge forces.
Don't be fooled into thinking that only solids are 'heavy' and that we can approximate everything as a volume-less particle. Fluids, such as liquids, have a density, exert an upward buoyancy on anything that should displace them, have increasing pressure with depth and are used in hydraulics to exert huge forces.
Key Concepts
Density is a material property (like Resistivity), which means that it is a constant value for a given material irrespective of its dimensions. The density of copper wire is the same as the density of a copper block.
Density is defined as the ratio of the mass of an object to its volume:
\(\rho={m\over V}\)
- \(\rho\) is density (kg m-3)
- \(m\) is mass (kg)
- \(V\) is volume (m-3)
Solids (especially metals) have a high density due to their high concentration of particles; copper's density is approximately 9000 kg m-3. Liquids come next; water has a density of 1000 kg m-3. Gases have a low density; air has a density of approximately 1 kg m-3 at sea level.
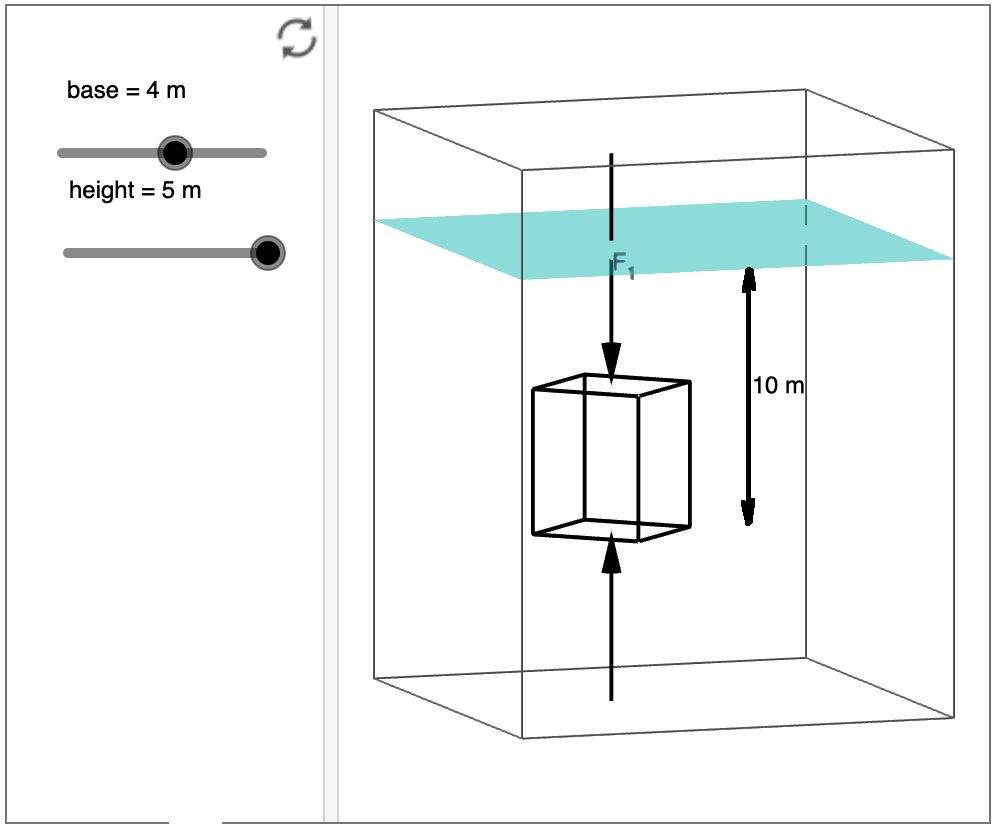
Archimedes' principle allows us to calculate the magnitude of fhe force of buoyancy. Buoyancy is equal to the weight of the fluid displaced, which we calculate using the fluid's density and volume (rather than mass):
\(B=\rho_f V_f g\)
- \(B\) is buoyancy (N)
- \(\rho_f\) is the density of the fluid (kg)
- \(V_f\) is the volume of fluid that is displaced by the object (m3)
- \(g\) is gravitational field strength (on earth, 9.81 N kg-1)
For an object that is fully submerged, the volume of the displaced fluid is equal to the volume of the object. For a floating object or an object that is partly submerged, only the volume of the object withn the fluid displaces fluid. Note that it is impossible for an object to float without displacing any fluid (however much polystyrene might appear to rest on top of a container of water!).
How much of AHL Static fluids have you understood?





 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn