
The Doppler effect is the first wave phenomenon that we consider in this Additional Higher Level topic. It is a change in frequency in observed sound caused by relative motion between the source, the observer or the medium. Here we will learn how to calculate these changes in frequency according to the type of relative motion, explain the impact of a sonic boom and discuss the equivalent effect for light.
Key Concepts
The Doppler effect is the change in frequency in observed sound due to relative motion between source and observer.
This is often observed when a car drives past sounding its horn. The frequency is increased when the car approaches because the car catches up with the waves, causing them to be squashed. The result is a reduction in wavelength, which leads to a higher frequency as a result of the wave equation:
\(f={c\over \lambda}\)
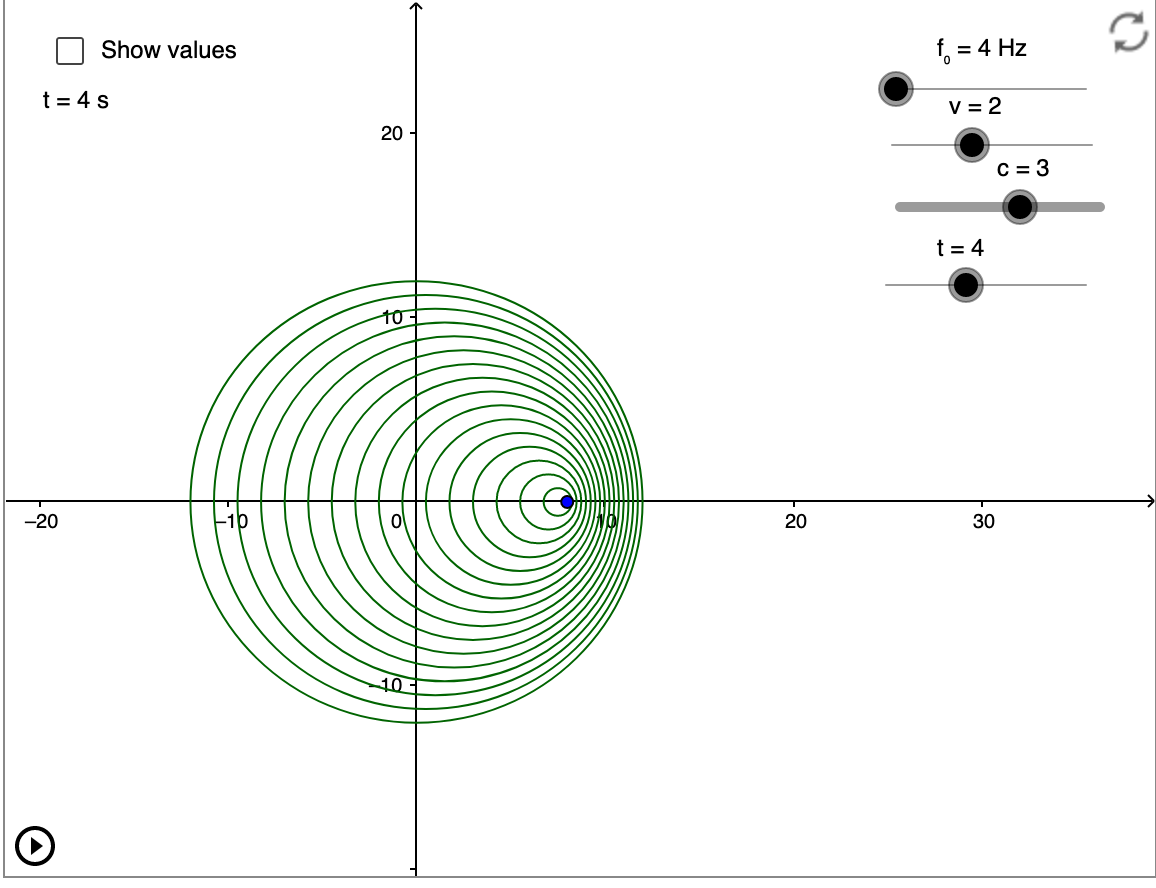
This diagram shows how you might sketch the Doppler effect.

Doppler shift is also experienced when an observer moves towards or away from a source that is stationary relative to the medium. This effect is simply due to the relative velocity between the observer and source.
The equation to calculate the observed frequency is amended as follows:
\(f'=f({v\pm u_o \over v})\)
- \(f'\) is the observed frequency
- \(f_0\) is the frequency emitted by the source
- \(v\) is the speed of sound (ms-1)
- \(u_o\) is the speed of the observer (ms-1), where \(u_o>0\) for the source moving towards the observer
This equation comes from use of the relative velocity of the sound as it approaches the observer, now \(v+u_o\) and using \(\lambda={v\over f}\):
\(f'={v+u_o\over \lambda}\Rightarrow f'=f{(v+u_o)\over v}\)
How much of Doppler effect have you understood?







 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn