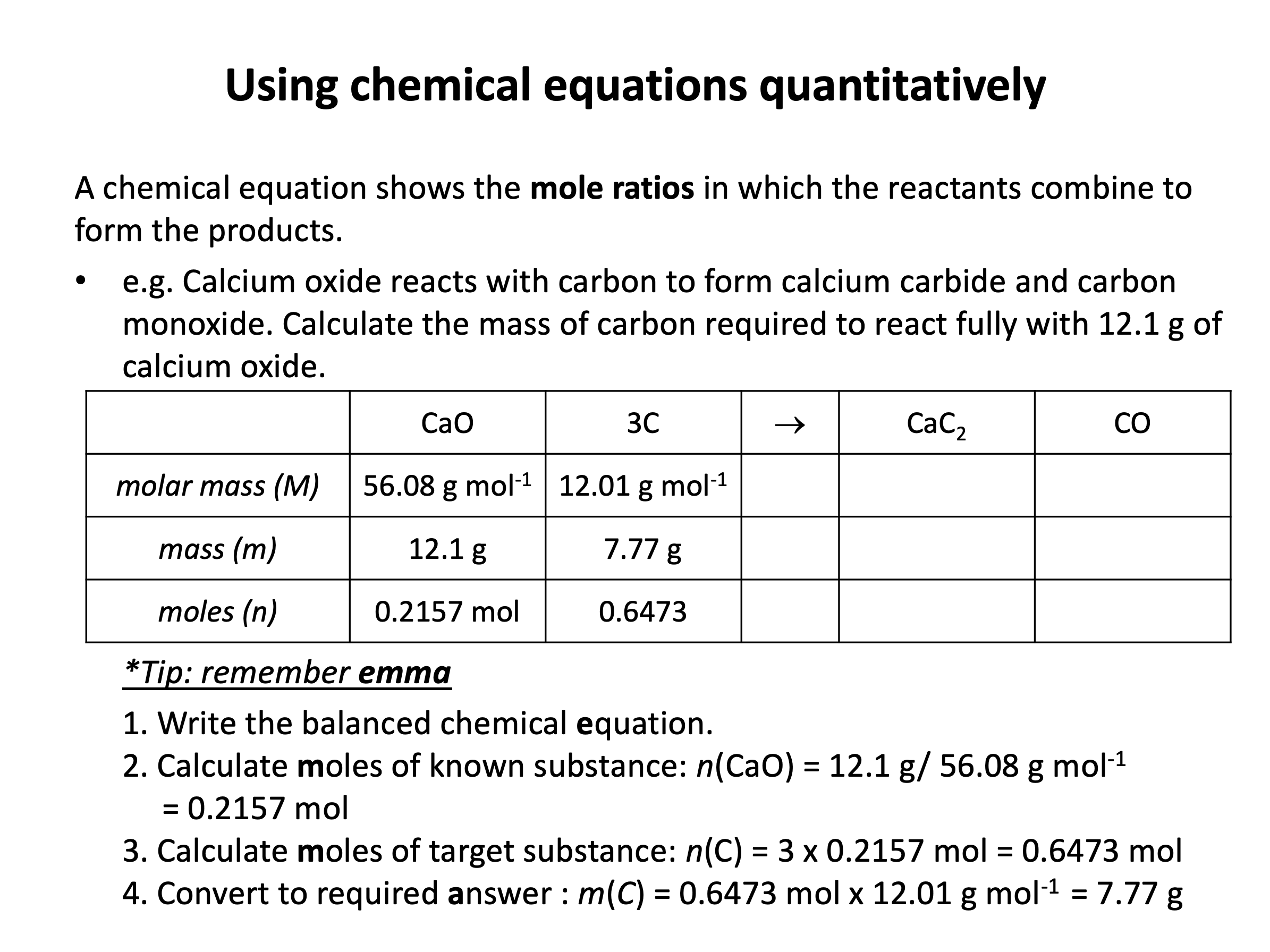
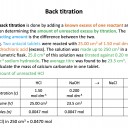
There is not a great deal of knowledge to learn in order to be confident with mole calculations, but you may well need lots of practice. There are only certain ways in which questions on stoichiometry are asked in exams, so attempt as many questions as you can until you start to recognise the different types and feel more confident answering them.
Remember that error carried forward is applied to when calculations are marked, so even if you make a mistake early in a question you can still score all the marks beyond! Use the table method in the revision cards to help you to organise your thoughts and your working.
Ensure you are confident using the terms below and learn the asterisked* definitions
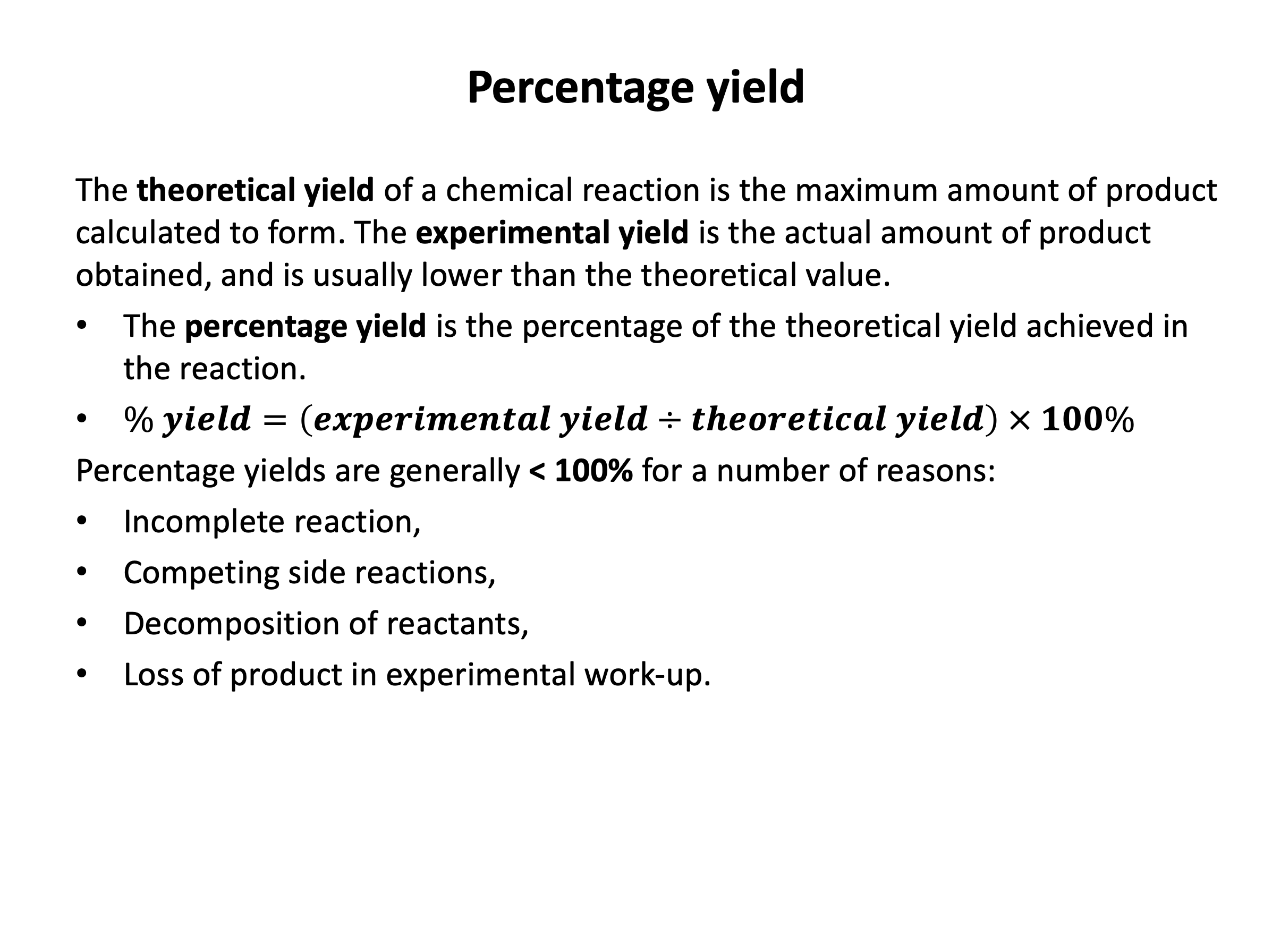
limiting reactant, excess reactant, theoretical yield, experimental yield, percentage yield, Avogadro's law*, ideal gas model, concentration, solution, standard solution
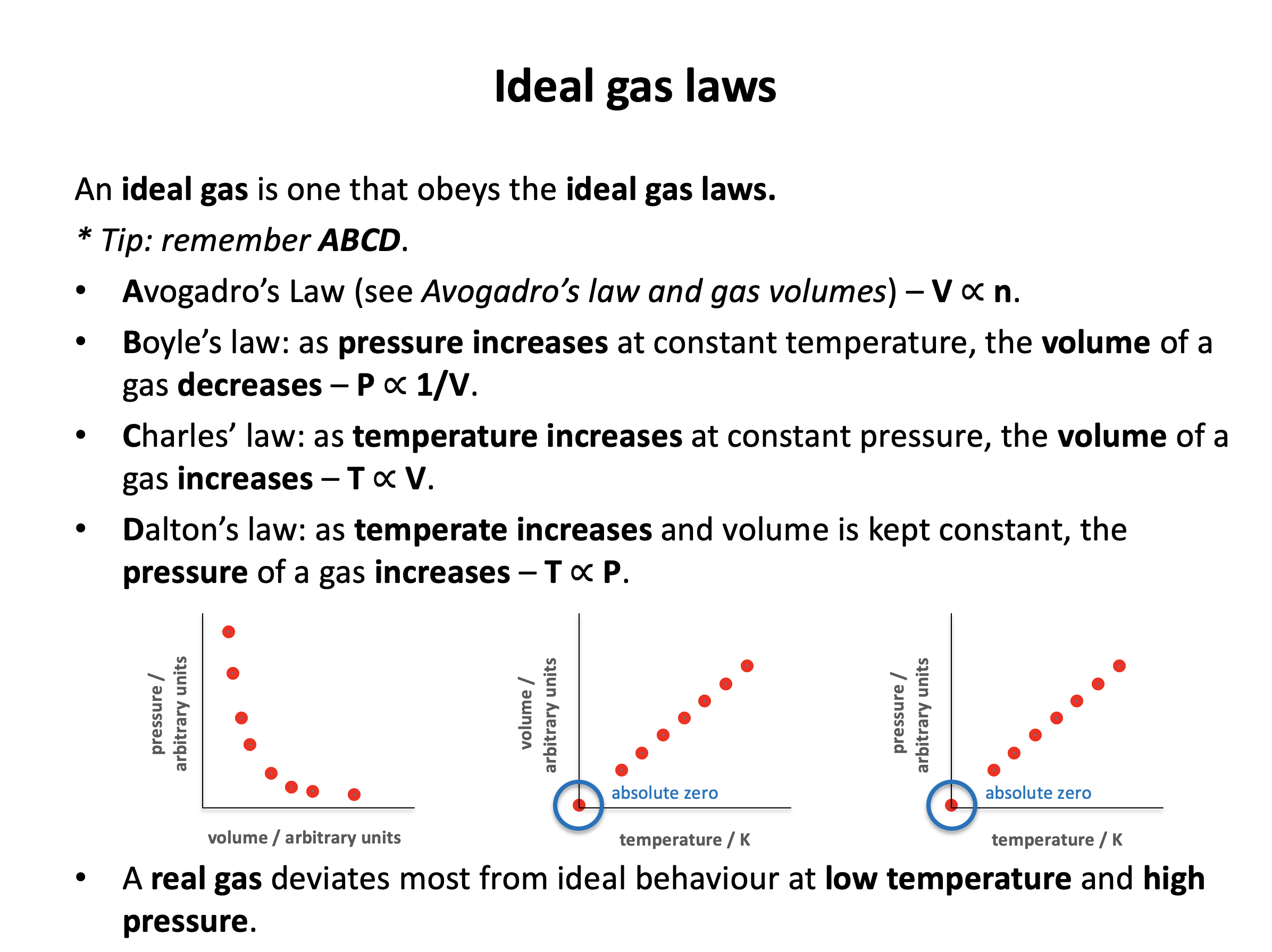
Which of the following are reasons why real gases deviate from the behaviour of ideal gases at, for example, very high pressures?
1: Real gases do not fill a container uniformly.
2: Real gases have intermolecular forces between the particles.
3: Real gases have volume associated with the particles.
Ideal gases are assumed to:
- be point particles with no volume;
- move in straight lines with random motion;
- behave as rigid spheres;
- engage in perfectly elastic collisions;
- have negligible forces between molecules.
A further assumption is that temperature represents a measure of the average kinetic energy of particles in a system.
Of the reasons given:
Real gases do fill a container uniformly, so reason 1 is not correct.
Real gases do have intermolecular forces between the particles and do have volume, so reasons 2 and 3 are correct in that they contribute to why real gases do not behave ideally at high pressures.
Thus 2 and 3 only is the correct answer.
5.00g of hex-1-ene was reacted with excess bromine at room temperature.
C6H12 + Br2 → C6H12Br2
Giving the answer to two decimal places, calculate what mass (g) of 1,2-dibromohexane should be formed?
Molar mass of C6H12 = (12.01×6)+(1.01×12) = 84.18
Molar mass of C6H12Br2 = 84.18 (above) + 79.90×2 = 243.92
Moles of hex-1-ene = mass / molar mass = 5.00 / 84.18 = 0.059396531
1:1 ratio of C6H12 to C6H12Br2, so moles of C6H12Br2 = 0.059396531
Mass of 1,2-dibromohexane = moles × molar mass = 0.059396531 × 243.92 = 14.4880019
Thus the answer is 14.49 (2 dp)
Incorrect answers:
14.51 can be obtained by using 12 and 1 as the molar masses of carbon and hydrogen respectively.
9.49 is the mass of bromine that will react with the hexene.
5.00 is the starting mass of the hexene re-stated.
Hydrogen and oxygen gases react together to produce water:
2H2(g) + O2(g) → 2H2O(l)
If 600cm3 of hydrogen and 500cm3 of oxygen are reacted to produce as much liquid water as possible, which gas/es will be left over at the end?
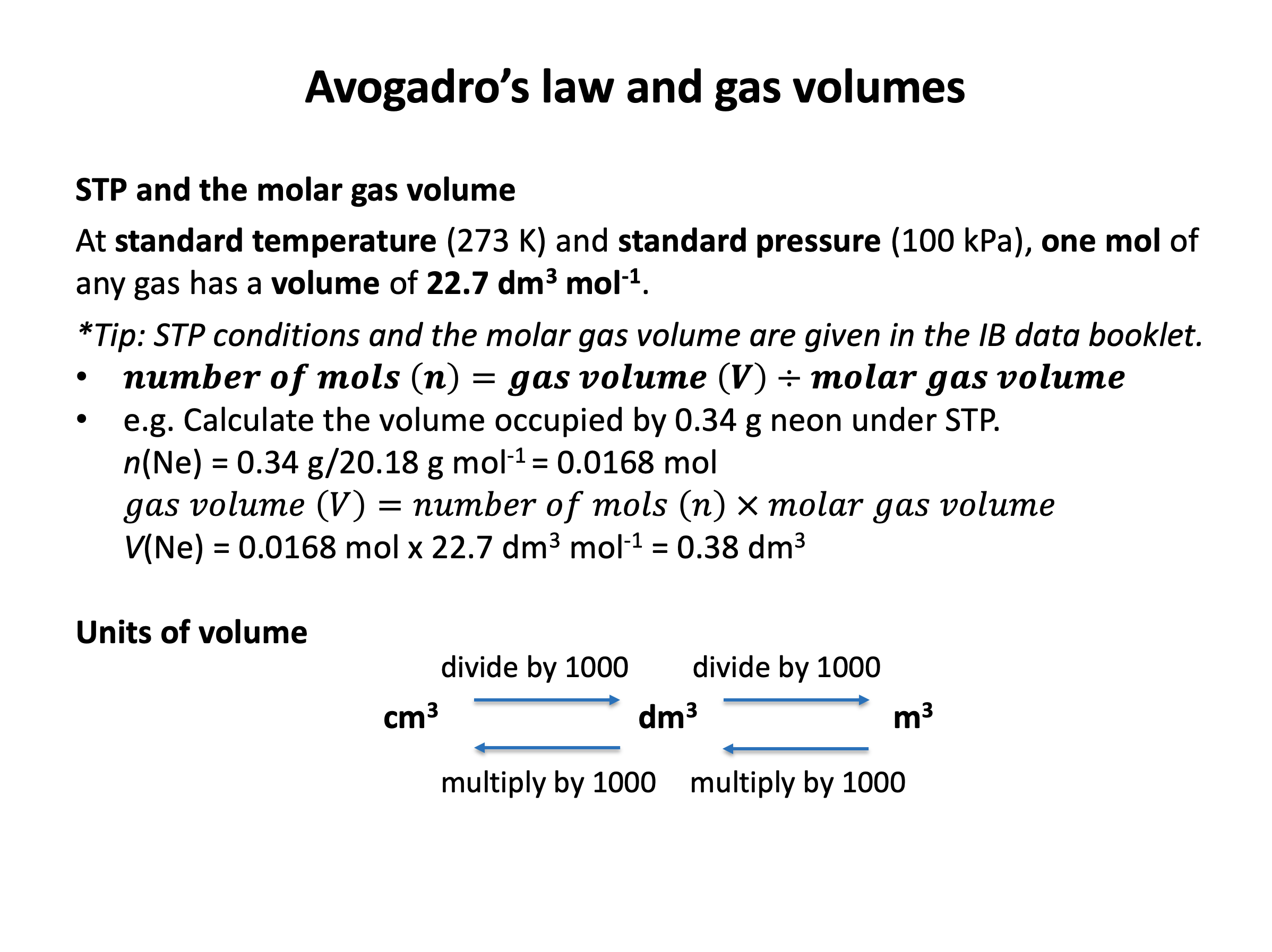
Ideal gases have a constant molar volume (at any given temperature and pressure).
The mole ratio here is 2H2 : 1O2. So oxygen will react with twice its volume of hydrogen (and vice versa, hydrogen will react with half its volume of oxygen).
To give the maximum amount of water, all 600cm3 of hydrogen will react with 300cm3 of oxygen. Oxygen gas is therefore in excess.
The amount of oxygen left over will be 500 − 300 = 200cm3
Thus the answer is 200cm3 of oxygen
Incorrect answers:
100cm3 hydrogen, assumes reacting in a 1:1 ratio (rather than 2:1).
400cm3 of hydrogen and 400cm3 of oxygen, suggests reacting in the correct ratio, but not producing a maximum amount of water.
None, assumes that all of the gases will react, which is incorrect.
A student carries out an experiment to make cyclohexene (C6H10) from cyclohexanol (C6H12O).
The student uses 6.50g of cyclohexanol and obtains 3.15g of cyclohexene.
What is the percentage yield of the experiment (in %)?
Molar masses:
C6H10 is (12.01×6)+(1.01×10) = 82.16
C6H12O is 82.16 (above) + (1.01×2)+16.00 = 100.18
The reaction has a 1:1 molar ratio.
moles of cyclohexanol = 6.50 / 100.18 = 0.06488321
theoretical yield of cyclohexene = moles × molar mass = 0.06488321 × 82.16 = 5.330804552g
experimental yield is given: 3.15g
percentage yield = (experimental yield / theorectical yield) × 100%
= (3.15 / 5.330804552) × 100% = 59.0905175%
Incorrect answers
5.33 is simply stating the theoretical yield (in g).
169 is assuming the percentage yield equation is inverted (5.33.../3.15).
40 assumes the molar masses are swapped around in the calculation (82.16 used instead of 100.18 and vice versa).
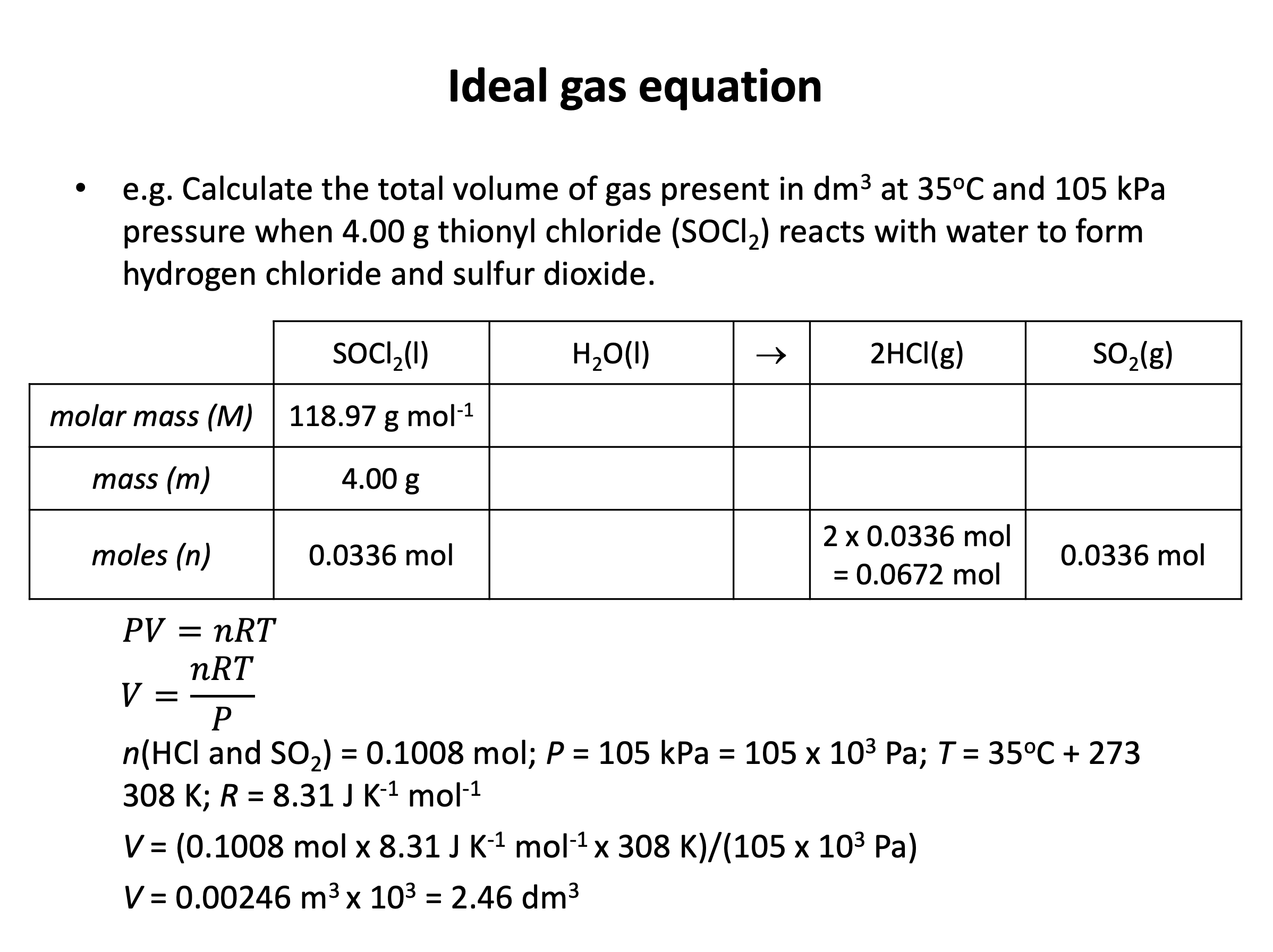
2.90g of a volatile liquid was evaporated and found to occupy approximately 1217cm3 at 20°C and atmospheric pressure (100kPa). To two significant figures, what is the molar mass of the volatile liquid?
Use PV=nRT (Gas constant, R, given in the data book as 8.31 J K−1 mol−1)
Units are critical; pressure in kPa and volume in dm3 work correctly (or Pa and m3) and temperature must be in Kelvin (°C + 273).
PV=nRT
100 × 1.217 = n × 8.31 × 293
n = 100 × 1.217 / 8.31 × 293 = 121.7 / 2434.83 = 0.05 mol (approximately)
molar mass = mass / mol
molar mass = 2.90 / 0.05 = 58 g mol−1
Thus 58 is the corect answer
Incorrect answers
4 assumes a temperature of 20 (rather than 293).
0.058 assumes a volume of 1217 (rather than 1.217).
0.0040 assumes both a temperature of 20 (rather than 293) and a volume of 1217 (rather than 1.217).
Lead nitrate solution and potassium iodide solution react when mixed to precipitate out lead iodide, a yellow solid, which can be filtered off:
Pb(NO3)2(aq) + 2KI(aq) → PbI2(s) + 2KNO3(aq)
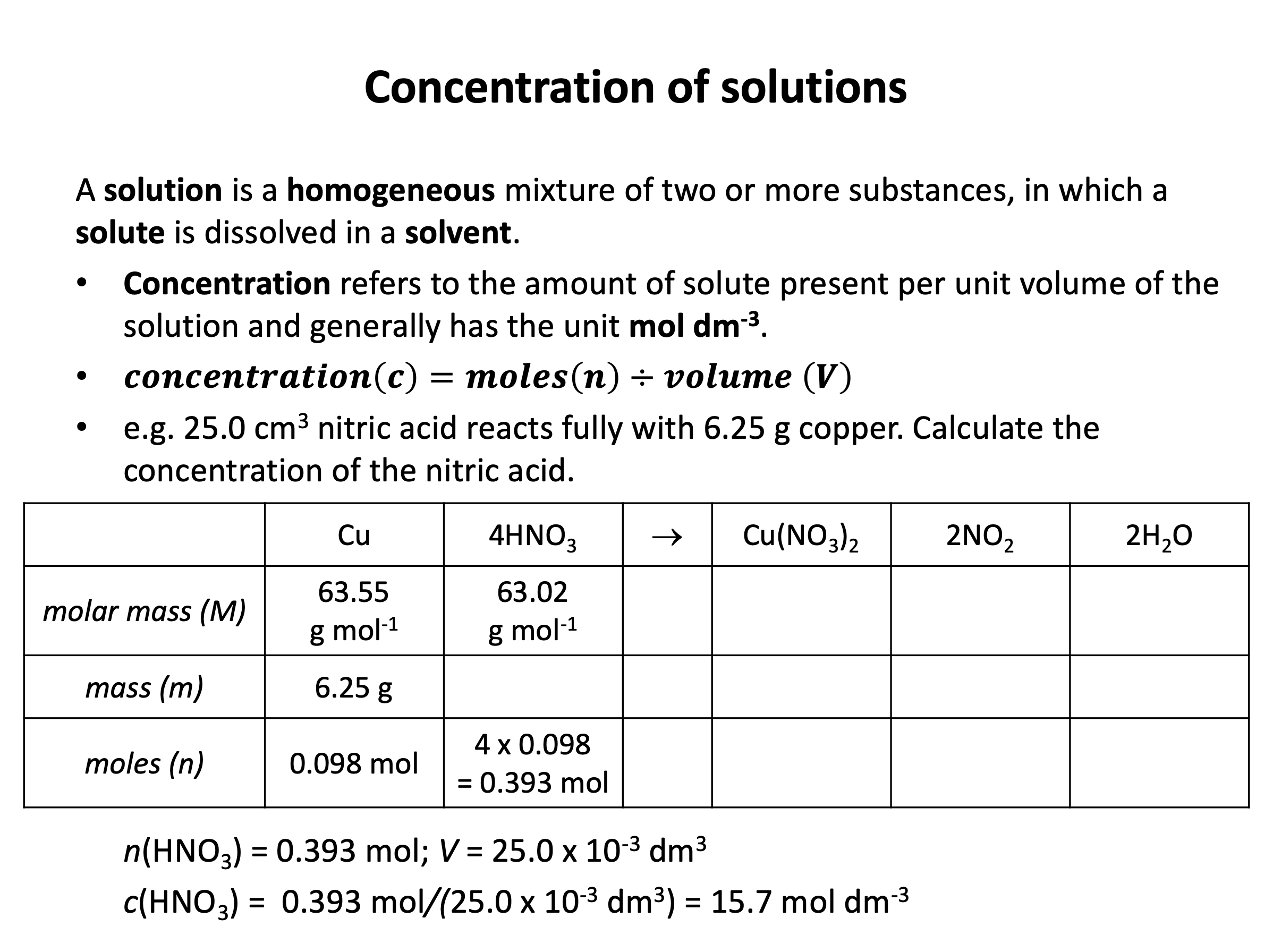
If 200cm3 of 0.100 mol dm−3 lead nitrate is added to 500cm3 of 0.200 mol dm−3 potassium iodide, what mass (in g) of lead iodide will be produced?
The quantities of both reactants are given, so this suggests that one reactant will be in excess.
The reaction has a 1:2 molar ratio of reactants.
moles = concentration × volume (dm3)
moles of lead nitrate = 0.100 × 0.200 = 0.0200mol
moles of potassium iodide = 0.200 × 0.500 = 0.100mol
potassium iodide is therefore in excess (×5 amount of lead nitrate, when only ×2 is needed to react completely).
All of the 0.0200mol of lead nitrate will react to produce 0.0200mol of lead iodide (1:1 ratio).
molar mass of lead iodide = 207.20 + (2×126.90) = 461.00
mass of lead iodide = moles × molar mass = 0.0200 × 461.00 = 9.22g
Thus the correct answer is 9.22
Incorrect answers
46.10 assumes 0.100mol of lead iodide is produced (rather than 0.0200).
6.68 assumes the molar mass of lead iodide uses a formula of PbI (rather than PbI2).
0.0200 states the moles of lead iodide (rather than the mass).
A titration can be used to measure the concentration of oxalic acid (ethandioic acid) in aqueous solution according to the following equation. Phenolphthalein is used as an indicator.
(COOH)2(aq) + 2NaOH(aq) → (COONa)2(aq) + 2H2O(l)
If an average of 21.50cm3 of 1.00 mol dm−3 sodium hydroxide is needed to neutralise 12.50cm3 of oxalic acid solution, what is the concentration of the oxalic acid? Give your answer in g dm−3.
The reaction has a 1:2 molar ratio of reactants.
moles = concentration × volume (dm3)
moles of sodium hydroxide = 1.00 × 0.02150 = 0.02150mol
moles of oxalic acid = 0.02150 ÷ 2 = 0.01075mol
concentration of oxalic acid = moles / volume (dm3)
= 0.01075 / 0.01250 = 0.8600 mol dm−3
molar mass of oxalic acid = (12.01 + 16.00 + 16.00 + 1.01) × 2 = 90.04
concentration of oxalic acid in g dm−3 = concentration in mol dm−3 × molar mass = 0.8600 × 90.04
= 77.4344 g dm−3
Thus the correct answer is 77.4
Incorrect answers
309.7 assumes moles of sodium hydroxide is ×2 instead of ÷2 (mole ratio the wrong way around).
38.7 assumes the molar mass of oxalic acid is half its actual value (the 2 in (COOH)2 has been ignored).
0.86 states the concentraion in mol dm−3 (rather than in g dm−3).
How much of Reacting masses and volumes have you understood?
Periodic table
Twitter 
Facebook 
LinkedIn