DP Mathematics: Applications and Interpretation Questionbank

Find the average number of visitors per concert in $2020$.

It is assumed that the concert hall will host $50$ concerts each year.

Use the average number of visitors per concert per year to predict the total number of people expected to attend the concert hall from when it opens until the end of $2025$.

Find the common ratio of the sequence.

Find the volume of the smallest slice of pie.

The apple pie has a volume of $61 425 {\text{cm}}^3$.

Find the total number of slices Mia can cut from this pie.

Find, $s$, in the form $s\left(\theta \right)=m\theta +c$.

After the enlargement scale factor equals zero, Ben continues to rotate the image for another two revolutions.

Describe the animation for these two revolutions, stating the final position of the sequence of squares.

state the coordinates, $\left(x_1, y_1\right)$, of its image in $U_1$.

show that ${\mathit{b}}_n=\left(\begin{array}{c}8\left(1-2^{-n}\right)\\ 8\left(1-2^{-n}\right)\end{array}\right)$.

hence find ${\mathit{b}}_1$.

Write down $E_{\theta }$.

Hence find the image of $(1, 1)$ after it is rotated $135°$ and enlarged.

Hence or otherwise, find the coordinates of the top left-hand corner in $U_7$.

Find the value of $\theta$ at which the enlargement scale factor equals zero.

Charlie ran on day $20$ of his fitness programme.

Daniella ran on day $20$ of her fitness programme.

Find how high the balloon will travel in the first $10$ minutes after it is launched.

The balloon is required to reach a height of at least $2520$ metres.

Determine whether it will reach this height.

Suggest a limitation of the given model.

Find the common ratio, $r$, for the sequence.

Find an expression for $u_n$.

Find the least value of $n$ such that $u_n<\frac{1}{2}$.

Calculate the total amount of acceptance fees paid to the university in the first $10$ years.

Calculate the percentage increase in applications from the first year to the second year.

Find $k$.

State whether, for all $n>k$, the university will have places available for all applicants. Justify your answer.

Write down the common ratio of the sequence.

Find the number of student applications the university expects to receive when $n=11$. Express your answer to the nearest integer.

Write down an expression for $v_n$ .

Show that the maximum height reached by the ball after it has bounced for the sixth time is $68 \text{cm}$, to the nearest $\text{cm}$.

Find the total vertical distance travelled by the ball from the point at which it is dropped until the fourth bounce.

Find the number of times, after the first bounce, that the maximum height reached is greater than $10 \text{cm}$.

The sum of an infinite geometric sequence is $9$.

The first term is $4$ more than the second term.

Find the third term. Justify your answer.

Describe what the value in part (d)(i) represents in this context.

Calculate the value of $\underset{n=1}{\overset{10}{\text{Σ}}}\left(625\times 1.{064}^{\left(n-1\right)}\right)$.

Write down the distance Rosa runs in the third training session;

Write down the distance Rosa runs in the $n$th training session.

Find the value of $k$.

Calculate the total distance, in kilometres, Rosa runs in the first 50 training sessions.

Find the distance Carlos runs in the fifth month of training.

Calculate the total distance Carlos runs in the first year.

Find the café’s profit during the 11th week.

Calculate the café’s total profit for the first 12 weeks.

Find the tea-shop’s profit during the 11th week.

Calculate the tea-shop’s total profit for the first 12 weeks.

In the mth week the tea-shop’s total profit exceeds the café’s total profit, for the first time since they both opened.

Find the value of m.

Write down the common ratio of the sequence.

Calculate, in CAD, the total amount John pays for the bicycle.

Find the value of the bicycle during the 5th year. Give your answer to two decimal places.

Calculate, in years, when the bicycle value will be less than 50 USD.

Find the total amount John has paid to insure his bicycle for the first 5 years.

Write down an equation, in terms of $u_1$ and $d$, for the amount of the drug that she receives on the seventh day.

Write down an equation, in terms of $u_1$ and $d$, for the amount of the drug that she receives on the eleventh day.

Write down the value of $d$ and the value of $u_1$.

Calculate the total amount of the drug, in mg, that she receives.

Find the amount of antibiotic, in mg, that Ted receives on the fifth day.

The daily amount of antibiotic Ted receives will first be less than 0.06 mg on the $k$ th day. Find the value of $k$.

Hence find the total amount of antibiotic, in mg, that Ted receives during the first $k$ days.

Find the common ratio.

Write down the second term of this sequence.

The sum of the first $k$ terms is greater than $2500$.

Find the smallest possible value of $k$.

Given that $q=8d^2$, find the other two real roots.

Find the common ratio of this sequence.

Find the common ratio.

Consider a geometric sequence where the first term is 768 and the second term is 576.

Find the least value of $n$ such that the $n$th term of the sequence is less than 7.

Find the sum to infinity of this sequence.

The following diagram shows [AB], with length 2 cm. The line is divided into an infinite number of line segments. The diagram shows the first three segments.

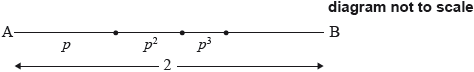
The length of the line segments are $p\text{ cm},p^2\text{ cm},p^3\text{ cm},\dots$, where .

Show that $p=\frac{2}{3}$.

Find the least value of n for which S_n > 163.

Show that $abc=8$.

Find the common ratio.

The following diagram shows [CD], with length $b\text{ cm}$, where $b>1$. Squares with side lengths $k\text{ cm},k^2\text{ cm},k^3\text{ cm},\dots$, where , are drawn along [CD]. This process is carried on indefinitely. The diagram shows the first three squares.

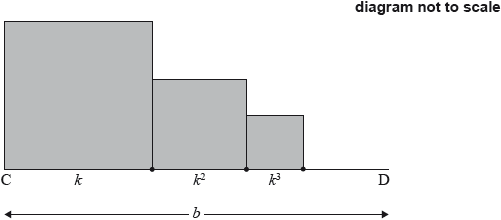
The total sum of the areas of all the squares is $\frac{9}{16}$. Find the value of $b$.

Show that one of the real roots is equal to 1.

Find the sum of the first 8 terms.