| Date | November 2017 | Marks available | 2 | Reference code | 17N.2.SL.TZ0.T_1 |
| Level | Standard Level | Paper | Paper 2 | Time zone | Time zone 0 |
| Command term | State | Question number | T_1 | Adapted from | N/A |
Question
A group of 800 students answered 40 questions on a category of their choice out of History, Science and Literature.
For each student the category and the number of correct answers, NN, was recorded. The results obtained are represented in the following table.
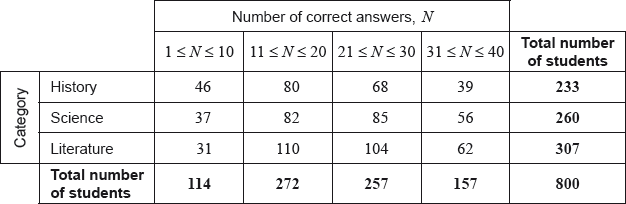
A χ2 test at the 5% significance level is carried out on the results. The critical value for this test is 12.592.
State whether N is a discrete or a continuous variable.
Write down, for N, the modal class;
Write down, for N, the mid-interval value of the modal class.
Use your graphic display calculator to estimate the mean of N;
Use your graphic display calculator to estimate the standard deviation of N.
Find the expected frequency of students choosing the Science category and obtaining 31 to 40 correct answers.
Write down the null hypothesis for this test;
Write down the number of degrees of freedom.
Write down the p-value for the test;
Write down the χ2 statistic.
State the result of the test. Give a reason for your answer.
Markscheme
* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.
discrete (A1)
[1 mark]
11⩽N⩽20 (A1)
[1 mark]
15.5 (A1)(ft)
Note: Follow through from part (b)(i).
[1 mark]
21.2 (21.2125) (G2)
[2 marks]
9.60 (9.60428…) (G1)
[1 marks]
260800×157800×800OR260×157800 (M1)
Note: Award (M1) for correct substitution into expected frequency formula.
=51.0 (51.025) (A1)(G2)
[2 marks]
choice of category and number of correct answers are independent (A1)
Notes: Accept “no association” between (choice of) category and number of correct answers. Do not accept “not related” or “not correlated” or “influenced”.
[1 mark]
6 (A1)
[1 mark]
0.0644 (0.0644123…) (G1)
[1 mark]
11.9 (11.8924…) (G2)
[2 marks]
the null hypothesis is not rejected (the null hypothesis is accepted) (A1)(ft)
OR
(choice of) category and number of correct answers are independent (A1)(ft)
as 11.9<12.592OR0.0644>0.05 (R1)
Notes: Award (R1) for a correct comparison of either their χ2 statistic to the χ2 critical value or their p-value to the significance level. Award (A1)(ft) from that comparison.
Follow through from part (f). Do not award (A1)(ft)(R0).
[2 marks]