| Date | November 2014 | Marks available | 2 | Reference code | 14N.1.hl.TZ0.7 |
| Level | HL only | Paper | 1 | Time zone | TZ0 |
| Command term | Find | Question number | 7 | Adapted from | N/A |
Question
Consider two functions f and g and their derivatives f′ and g′. The following table shows the values for the two functions and their derivatives at x=1, 2 and 3.
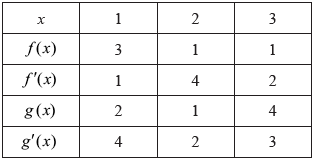
Given that p(x)=f(x)g(x) and h(x)=g∘f(x), find
p′(3);
h′(2).
Markscheme
p′(3)=f′(3)g(3)+g′(3)f(3) (M1)
Note: Award M1 if the derivative is in terms of x or 3.
=2×4+3×1
=11 A1
[2 marks]
h′(x)=g′(f(x))f′(x) (M1)(A1)
h′(2)=g′(1)f′(2) A1
=4×4
=16 A1
[4 marks]
Total [6 marks]
Examiners report
This was a problem question for many candidates. Some quite strong candidates, on the evidence of their performance on other questions, did not realise that ‘composite functions’ and ‘functions of a function’ were the same thing, and therefore that the chain rule applied.
This was a problem question for many candidates. Some quite strong candidates, on the evidence of their performance on other questions, did not realise that ‘composite functions’ and ‘functions of a function’ were the same thing, and therefore that the chain rule applied.

