| Date | May 2014 | Marks available | 2 | Reference code | 14M.1.hl.TZ1.11 |
| Level | HL only | Paper | 1 | Time zone | TZ1 |
| Command term | Show that | Question number | 11 | Adapted from | N/A |
Question
Consider the function f(x)=lnxx, x>0.
The sketch below shows the graph of y= f(x) and its tangent at a point A.
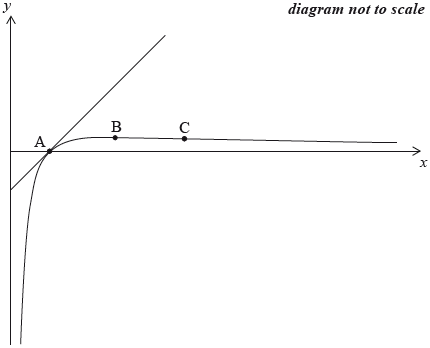
Show that f′(x)=1−lnxx2.
Find the coordinates of B, at which the curve reaches its maximum value.
Find the coordinates of C, the point of inflexion on the curve.
The graph of y= f(x) crosses the x-axis at the point A.
Find the equation of the tangent to the graph of f at the point A.
The graph of y= f(x) crosses the x-axis at the point A.
Find the area enclosed by the curve y=f(x), the tangent at A, and the line x=e.
Markscheme
f′(x)=x×1x−lnxx2 M1A1
=1−lnxx2 AG
[2 marks]
1−lnxx2=0 has solution x=e M1A1
y=1e A1
hence maximum at the point (e, 1e)
[3 marks]
f″ M1A1
= \frac{{2\ln x - 3}}{{{x^3}}}
Note: The M1A1 should be awarded if the correct working appears in part (b).
point of inflexion where f''(x) = 0 M1
so x = {{\text{e}}^{\frac{3}{2}}},{\text{ }}y = \frac{3}{2}{{\text{e}}^{\frac{{ - 3}}{2}}} A1A1
C has coordinates \left( {{{\text{e}}^{\frac{3}{2}}},{\text{ }}\frac{3}{2}{{\text{e}}^{\frac{{ - 3}}{2}}}} \right)
[5 marks]
f(1) = 0 A1
f'(1) = 1 (A1)
y = x + c (M1)
through (1, 0)
equation is y = x - 1 A1
[4 marks]
METHOD 1
area = \int_1^{\text{e}} {x - 1 - \frac{{\ln x}}{x}{\text{d}}x} M1A1A1
Note: Award M1 for integration of difference between line and curve, A1 for correct limits, A1 for correct expressions in either order.
\int {\frac{{\ln x}}{x}{\text{d}}x = \frac{{{{(\ln x)}^2}}}{2}} ( + c) (M1)A1
\int {(x - 1){\text{d}}x = \frac{{{x^2}}}{2} - x( + c)} A1
= \left[ {\frac{1}{2}{x^2} - x - \frac{1}{2}{{(\ln x)}^2}} \right]_1^{\text{e}}
= \left( {\frac{1}{2}{{\text{e}}^2} - {\text{e}} - \frac{1}{2}} \right) - \left( {\frac{1}{2} - 1} \right)
= \frac{1}{2}{{\text{e}}^2} - {\text{e}} A1
METHOD 2
area = area of triangle - \int_1^e {\frac{{\ln x}}{x}{\text{d}}x} M1A1
Note: A1 is for correct integral with limits and is dependent on the M1.
\int {\frac{{\ln x}}{x}{\text{d}}x = \frac{{{{(\ln x)}^2}}}{2}( + c)} (M1)A1
area of triangle = \frac{1}{2}(e - 1)(e - 1) M1A1
\frac{1}{2}(e - 1)(e - 1) - \left( {\frac{1}{2}} \right) = \frac{1}{2}{{\text{e}}^2} - {\text{e}} A1
[7 marks]

