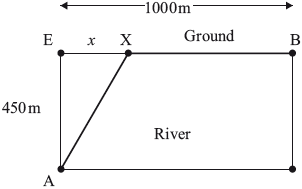
Engineers need to lay pipes to connect two cities A and B that are separated by a river of width 450 metres as shown in the following diagram. They plan to lay the pipes under the river from A to X and then under the ground from X to B. The cost of laying the pipes under the river is five times the cost of laying the pipes under the ground.

Let ${\text{EX}} = x$.

 

Let k be the cost, in dollars per metre, of laying the pipes under the ground.

(a)     Show that the total cost C, in dollars, of laying the pipes from A to B is given by $C = 5k\sqrt {202\,500 + {x^2}}  + (1000 - x)k$.

(b)     (i)     Find $\frac{{{\text{d}}C}}{{{\text{d}}x}}$.

          (ii)     Hence find the value of x for which the total cost is a minimum, justifying that this value is a minimum.

(c)     Find the minimum total cost in terms of k.

The angle at which the pipes are joined is ${\rm{A\hat XB}} = \theta$.

(d)     Find $\theta$ for the value of x calculated in (b).

For safety reasons $\theta$ must be at least 120°.

Given this new requirement,

(e)     (i)     find the new value of x which minimises the total cost;

          (ii)     find the percentage increase in the minimum total cost.

(a)     $C = {\text{AX}} \times 5k + {\text{XB}} \times k$     (M1)

 

Note:     Award (M1) for attempting to express the cost in terms of AX, XB and k.

 

$= 5k\sqrt {{{450}^2} + {x^2}}  + (1000 - x)k$     A1

$= 5k\sqrt {202\,500 + {x^2}}  + (1000 - x)k$     AG

[2 marks]

 

(b)     (i)     $\frac{{{\text{d}}C}}{{{\text{d}}x}} = k\left[ {\frac{{5 \times 2x}}{{2\sqrt {202\,500 + {x^2}} }} - 1} \right] = k\left( {\frac{{5x}}{{\sqrt {202\,500 + {x^2}} }} - 1} \right)$     M1A1

 

Note:     Award M1 for an attempt to differentiate and A1 for the correct derivative.

 

          (ii)     attempting to solve $\frac{{{\text{d}}C}}{{{\text{d}}x}} = 0$     M1

          $\frac{5}{{\sqrt {202\,500 + {x^2}} }} = 1$     (A1)

          $x = 91.9{\text{ (m) }}\left( { = \frac{{75\sqrt 6 }}{2}{\text{ (m)}}} \right)$     A1

          METHOD 1

          for example,

          at $x = 91\frac{{{\text{d}}C}}{{{\text{d}}x}} =  - 0.00895k < 0$     M1

          at $x = 92\frac{{{\text{d}}C}}{{{\text{d}}x}} =  0.001506k > 0$     A1

 

Note:     Award M1 for attempting to find the gradient either side of $x = 91.9$ and A1 for two correct values.

 

          thus $x = 91.9$ gives a minimum     AG

          METHOD 2

          $\frac{{{{\text{d}}^2}C}}{{{\text{d}}{x^2}}} = \frac{{1\,012\,500k}}{{{{\left( {{x^2} + 202\,500} \right)}^{\frac{3}{2}}}}}$

          at $x = 91.9\frac{{{{\text{d}}^2}C}}{{{\text{d}}{x^2}}} = 0.010451k > 0$     (M1)A1

 

Note:     Award M1 for attempting to find the second derivative and A1 for the correct value.

 

Note:     If $\frac{{{{\text{d}}^2}C}}{{{\text{d}}{x^2}}}$ is obtained and its value at $x = 91.9$ is not calculated, award (M1)A1 for correct reasoning eg, both numerator and denominator are positive at $x = 91.9$.

 

          thus $x = 91.9$ gives a minimum     AG

          METHOD 3

          Sketching the graph of either C versus x or $\frac{{{\text{d}}C}}{{{\text{d}}x}}$ versus x.     M1

          Clearly indicating that $x = 91.9$ gives the minimum on their graph.     A1

[7 marks]

 

(c)     ${C_{\min }} = 3205k$     A1

 

Note:     Accept 3200k.

     Accept 3204k.

 

[1 mark]

 

(d)     $\arctan \left( {\frac{{450}}{{91.855865{\text{K}}}}} \right) = 78.463{\text{K}}^\circ$     M1

$180 - 78.463{\text{K = 101.537K}}$

$= 102^\circ$     A1

[2 marks]

 

(e)     (i)     when $\theta  = 120^\circ ,{\text{ }}x = 260{\text{ (m) }}\left( {\frac{{450}}{{\sqrt 3 }}{\text{ (m)}}} \right)$     A1

          (ii)     $\frac{{133.728{\text{K}}}}{{3204.5407685{\text{K}}}} \times 100\%$     M1

          $= 4.17{\text{ (% )}}$     A1

[3 marks]

 

Total [15 marks]

[N/A]