| Date | May 2014 | Marks available | 6 | Reference code | 14M.1.hl.TZ1.6 |
| Level | HL only | Paper | 1 | Time zone | TZ1 |
| Command term | Sketch and State | Question number | 6 | Adapted from | N/A |
Question
The first set of axes below shows the graph of y= f(x) for −4⩽x⩽4.
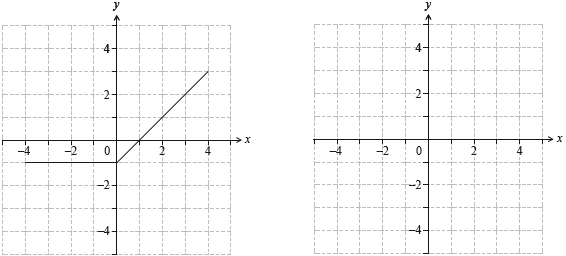
Let g(x)=∫x−4f(t)dt for −4⩽x⩽4.
(a) State the value of x at which g(x) is a minimum.
(b) On the second set of axes, sketch the graph of y=g(x).
Markscheme
(a) x=1 A1
[1 mark]
(b) A1 for point (–4, 0)
A1 for (0, − 4)
A1 for min at x=1 in approximately the correct place
A1 for (4, 0)
A1 for shape including continuity at x=0
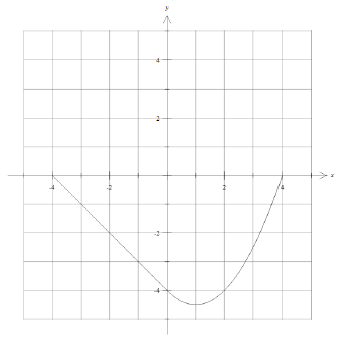
[5 marks]
Total [6 marks]
Examiners report
[N/A]
Syllabus sections
Topic 6 - Core: Calculus » 6.5 » Area of the region enclosed by a curve and the x-axis or y-axis in a given interval; areas of regions enclosed by curves.
Show 31 related questions

