| Date | November 2017 | Marks available | 4 | Reference code | 17N.3dm.hl.TZ0.3 |
| Level | HL only | Paper | Paper 3 Discrete mathematics | Time zone | TZ0 |
| Command term | Prove that | Question number | 3 | Adapted from | N/A |
Question
Consider κn, a complete graph with n vertices, n⩾2. Let T be a fixed spanning tree of κn.
Draw the complete bipartite graph κ3,3.
Prove that κ3,3 is not planar.
A connected graph G has v vertices. Prove, using Euler’s relation, that a spanning tree for G has v−1 edges.
If an edge E is chosen at random from the edges of κn, show that the probability that E belongs to T is equal to 2n.
Markscheme
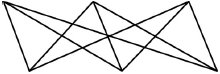 A1
A1
[1 mark]
assume κ3,3 is planar
κ3,3 has no cycles of length 3 R1
use of e⩽2v−4 M1
e=9 and v=6 A1
hence inequality not satisfied 9 ⩽̸ 8 R1
so κ3,3 is not planar AG
Note: use of e⩽3v−6 with e=9 and v=6 and concluding that this inequality does not show whether κ3,3 is planar or not just gains R1.
[4 marks]
a spanning tree (is planar and) has one face A1
Euler’s relation is v−e+f=2
v−e+1=2 M1
⇒e=v−1 AG
[2 marks]
κn has (n2) edges (n(n−1)2 edges) (A1)
P(E belongs to T)=n−1(n(n−1)2) M1A1
clear evidence of simplification of the above expression M1
=2n AG
[4 marks]

