| Date | November 2017 | Marks available | 2 | Reference code | 17N.1.hl.TZ0.9 |
| Level | HL only | Paper | 1 | Time zone | TZ0 |
| Command term | Find | Question number | 9 | Adapted from | N/A |
Question
In the following diagram, →OA = a, →OB = b. C is the midpoint of [OA] and →OF=16→FB.
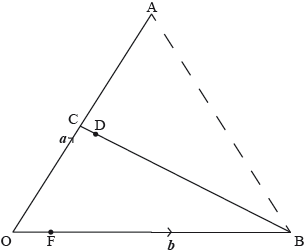
It is given also that →AD=λ→AF and →CD=μ→CB, where λ, μ∈R.
Find, in terms of a and b →OF.
Find, in terms of a and b →AF.
Find an expression for →OD in terms of a, b and λ;
Find an expression for →OD in terms of a, b and μ.
Show that μ=113, and find the value of λ.
Deduce an expression for →CD in terms of a and b only.
Given that area ΔOAB=k(area ΔCAD), find the value of k.
Markscheme
→OF=17b A1
[1 mark]
→AF=→OF−→OA (M1)
=17b – a A1
[2 marks]
→OD= a +λ(17b−a) (=(1−λ)a+λ7b) M1A1
[2 marks]
→OD=12 a +μ(−12a+b) (=(12−μ2)a+μb) M1A1
[2 marks]
equating coefficients: M1
λ7=μ, 1−λ=1−μ2 A1
solving simultaneously: M1
λ=713, μ=113 A1AG
[4 marks]
→CD=113→CB
=113(b−12a) (=−126a+113b) M1A1
[2 marks]
METHOD 1
area ΔACD=12CD×AC×sinAˆCB (M1)
area ΔACB=12CB×AC×sinAˆCB (M1)
ratio area ΔACDarea ΔACB=CDCB=113 A1
k=area ΔOABarea ΔCAD=13area ΔCAB×area ΔOAB (M1)
=13×2=26 A1
METHOD 2
area ΔOAB=12|a×b| A1
area ΔCAD=12|→CA×→CD| or 12|→CA×→AD| M1
=12|12a×(−126a+113b)|
=12|12a×(−126a)+12a×113b| (M1)
=12×12×113|a×b| (=152|a×b|) A1
area ΔOAB=k(area ΔCAD)
12|a×b|=k152|a×b|
k=26 A1
[5 marks]

