The folium of Descartes is a curve defined by the equation ${x^3} + {y^3} - 3xy = 0$, shown in the following diagram.

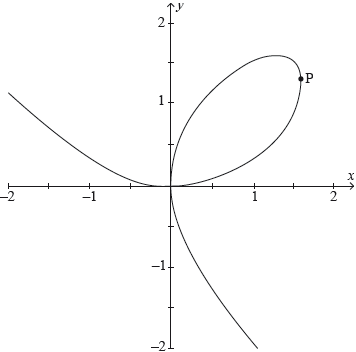
Determine the exact coordinates of the point P on the curve where the tangent line is parallel to the $y$-axis.

${x^3} + {y^3} - 3xy = 0$

$3{x^2} + 3{y^2}\frac{{{\text{d}}y}}{{{\text{d}}x}} - 3x\frac{{{\text{d}}y}}{{{\text{d}}x}} - 3y = 0$     M1A1

Note:     Differentiation wrt $y$ is also acceptable.

$\frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{{3y - 3{x^2}}}{{3{y^2} - 3x}}{\text{ }}\left( { = \frac{{y - {x^2}}}{{{y^2} - x}}} \right)$     (A1)

Note:     All following marks may be awarded if the denominator is correct, but the numerator incorrect.

${y^2} - x = 0$     M1

EITHER

$x = {y^2}$

${y^6} + {y^3} - 3{y^3} = 0$     M1A1

${y^6} - 2{y^3} = 0$

${y^3}({y^3} - 2) = 0$

$(y \ne 0)\therefore y = \sqrt[3]{2}$     A1

$x = {\left( {\sqrt[3]{2}} \right)^2}{\text{ }}\left( { = \sqrt[3]{4}} \right)$     A1

OR

${x^3} + xy - 3xy = 0$     M1

$x({x^2} - 2y) = 0$

$x \ne 0 \Rightarrow y = \frac{{{x^2}}}{2}$     A1

${y^2} = \frac{{{x^4}}}{4}$

$x = \frac{{{x^4}}}{4}$

$x({x^3} - 4) = 0$

$(x \ne 0)\therefore x = \sqrt[3]{4}$     A1

$y = \frac{{{{\left( {\sqrt[3]{4}} \right)}^2}}}{2} = \sqrt[3]{2}$     A1

[8 marks]