| Date | May 2017 | Marks available | 2 | Reference code | 17M.2.hl.TZ2.4 |
| Level | HL only | Paper | 2 | Time zone | TZ2 |
| Command term | Find | Question number | 4 | Adapted from | N/A |
Question
Find the set of values of k that satisfy the inequality k2−k−12<0.
[2]
a.
The triangle ABC is shown in the following diagram. Given that cosB<14, find the range of possible values for AB.
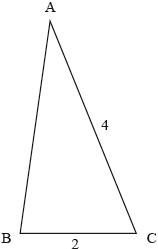
[4]
b.
Markscheme
k2−k−12<0
(k−4)(k+3)<0 (M1)
−3<k<4 A1
[2 marks]
a.
cosB=22+c2−424c (or 16=22+c2−4ccosB) M1
⇒c2−124c<14 A1
⇒c2−c−12<0
from result in (a)
0<AB<4 or −3<AB<4 (A1)
but AB must be at least 2
⇒2<AB<4 A1
Note: Allow ⩽ for either of the final two A marks.
[4 marks]
b.
Examiners report
[N/A]
a.
[N/A]
b.

