| Date | November 2016 | Marks available | 3 | Reference code | 16N.3sp.hl.TZ0.1 |
| Level | HL only | Paper | Paper 3 Statistics and probability | Time zone | TZ0 |
| Command term | Find | Question number | 1 | Adapted from | N/A |
Question
In this question you may assume that these data are a random sample from a bivariate normal distribution, with population product moment correlation coefficient \(\rho \).
Richard wishes to do some research on two types of exams which are taken by a large number of students. He takes a random sample of the results of 10 students, which are shown in the following table.
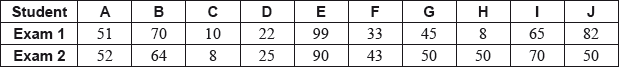
Using these data, it is decided to test, at the 1% level, the null hypothesis \({H_0}:\rho = 0\) against the alternative hypothesis \({H_1}:\rho > 0\).
Richard decides to take the exams himself. He scored 11 on Exam 1 but his result on Exam 2 was lost.
Caroline believes that the population mean mark on Exam 2 is 6 marks higher than the population mean mark on Exam 1. Using the original data from the 10 students, it is decided to test, at the 5% level, this hypothesis against the alternative hypothesis that the mean of the differences, \({\text{d}} = {\text{exam 2 mark }} - {\text{ exam 1 mark}}\), is less than 6 marks.
For these data find the product moment correlation coefficient, \(r\).
(i) State the distribution of the test statistic (including any parameters).
(ii) Find the \(p\)-value for the test.
(iii) State the conclusion, in the context of the question, with the word “correlation” in your answer. Justify your answer.
Using a suitable regression line, find an estimate for his score on Exam 2, giving your answer to the nearest integer.
(i) State the distribution of your test statistic (including any parameters).
(ii) Find the \(p\)-value.
(iii) State the conclusion, justifying the answer.
Markscheme
\(r = 0.804\) A2
Note: Accept any number that rounds to 0.80.
[2 marks]
(i) \(t\) distribution with 8 degrees of freedom A1A1
(ii) \(p{\text{ - value}} = 0.00254\) A2
Notes: Accept any number that rounds to 0.0025.
Award A1 for 2-tail test giving an answer that rounds to 0.0051.
(iii) \(p{\text{ - value}} < 0.01\), so conclude that there is positive correlation R1A1
Notes: Only award the A1 if the R1 is awarded.
Do not accept just “reject \({H_0}\)” or “accept \({H_1}\)”.
The words “positive correlation” must be seen.
[6 marks]
regression line of \(y\) (Exam 2 mark) on \(x\) (Exam 1 mark) is (M1)
\(y = 0.59407 \ldots x + 21.387 \ldots \) (A1)
\(x = 11\) gives \(y = 28\) (to nearest integer) A1
[3 marks]
(i) applying the \(t\) test to the differences
\(t\) distribution with 9 degrees of freedom A1A1
(ii) \(p{\text{ - value}} = 0.239\) A2
Notes: Accept any number that rounds to 0.24.
Award A1 if subtraction done the wrong way round giving \(p{\text{ - value}} = 0.109\).
(iii) \(p{\text{ - value}} > 0.05\), so accept \({H_0}\) or \({u_d} = 6\) R1A1
[6 marks]
